Question 9:
Solution by scale drawing is not accepted.
Diagram 5 shows the path of a moving point P(x, y). P always moves at a constant distance from point A.
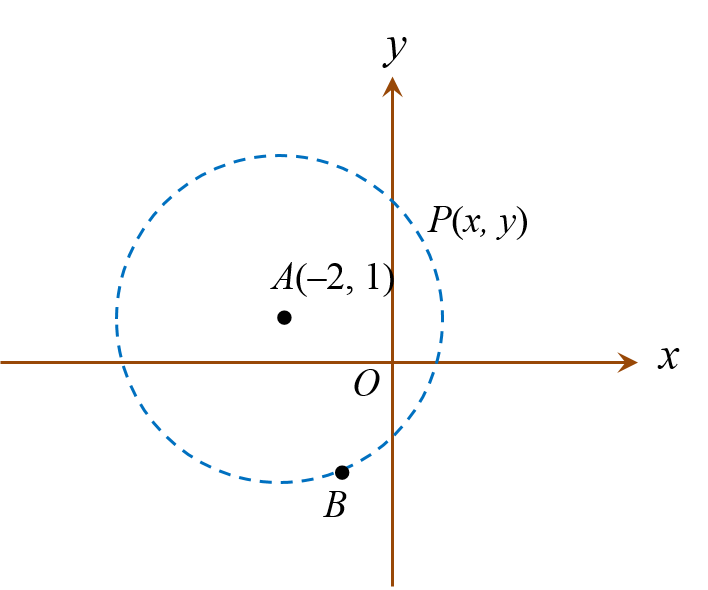 Diagram 5
Diagram 5
B(–1, –2) and R(–5, q) lie on path of point P. The straight line BC is a tangent to the path and
intersects the x-axis at point C.
Find
(a) the equation of the path of point P, [3 marks]
(b) the possible values of q, [2 marks]
(c) the area of ∆ ABC. [5 marks]
Solution:
(a)
Radius AB=√(−2+1)2+(1+2)2 =√1+9 =√10PA=√10√(x+2)2+(y−1)2=√10(x+2)2+(y−1)2=10x2+4x+4+y2−2y+1−10=0x2+y2+4x−2y−5=0
(b)
When x = –5, y = q,
(–5)2 + q2 + 4(–5) – 2q – 5 = 0
25 + q2 – 20 – 2q – 5 = 0
q2 – 2q = 0
q(q – 2) = 0
q = 0, q = 2
(c)
mAB×mBC=−1(1+2−2+1)×mBC=−1(3−1)×mBC=−1mBC=13Equation of BC:y−(−2)=13(x+1)3(y+2)=x+13y+6=x+13y=x−5At point C, y=0x−5=0x=5C=(5, 0)
Area of ΔABC=12| 5 −2 −1 5 0 1 −2 0|=12|5+4+0−0−(−1)−(−10)|=12|20|=10 unit2
Solution by scale drawing is not accepted.
Diagram 5 shows the path of a moving point P(x, y). P always moves at a constant distance from point A.
 Diagram 5
Diagram 5B(–1, –2) and R(–5, q) lie on path of point P. The straight line BC is a tangent to the path and
intersects the x-axis at point C.
Find
(a) the equation of the path of point P, [3 marks]
(b) the possible values of q, [2 marks]
(c) the area of ∆ ABC. [5 marks]
Solution:
(a)
Radius AB=√(−2+1)2+(1+2)2 =√1+9 =√10PA=√10√(x+2)2+(y−1)2=√10(x+2)2+(y−1)2=10x2+4x+4+y2−2y+1−10=0x2+y2+4x−2y−5=0
(b)
When x = –5, y = q,
(–5)2 + q2 + 4(–5) – 2q – 5 = 0
25 + q2 – 20 – 2q – 5 = 0
q2 – 2q = 0
q(q – 2) = 0
q = 0, q = 2
(c)
mAB×mBC=−1(1+2−2+1)×mBC=−1(3−1)×mBC=−1mBC=13Equation of BC:y−(−2)=13(x+1)3(y+2)=x+13y+6=x+13y=x−5At point C, y=0x−5=0x=5C=(5, 0)
Area of ΔABC=12| 5 −2 −1 5 0 1 −2 0|=12|5+4+0−0−(−1)−(−10)|=12|20|=10 unit2