Question 7 (10 marks):
(a) Prove sin(3x+π6)−sin(3x−π6)=cos3x(b) Hence,(i) solve the equation sin(3x2+π6)−sin(3x2−π6)=12 for 0≤x≤2π and give your answer in the simplest fraction form in terms of π radian.(ii) sketch the graph of y=sin(3x+π6)−sin(3x−π6)−12 for 0≤x≤π.
Solution:
(a) Left hand side,sin(3x+π6)−sin(3x−π6)=[sin3xcosπ6+cos3xsinπ6]−[sin3xcosπ6−cos3xsinπ6]=2[cos3xsinπ6]=2[cos3x(12)]=cos3x(right hand side)
(b)(i)sin(3x2+π6)−sin(3x2−π6)=12,0≤x≤2πcos3x2=123x2=π3,(2π−π3),(2π+π3)3x2=π3,5π3,7π3x=2π9,10π9,14π9
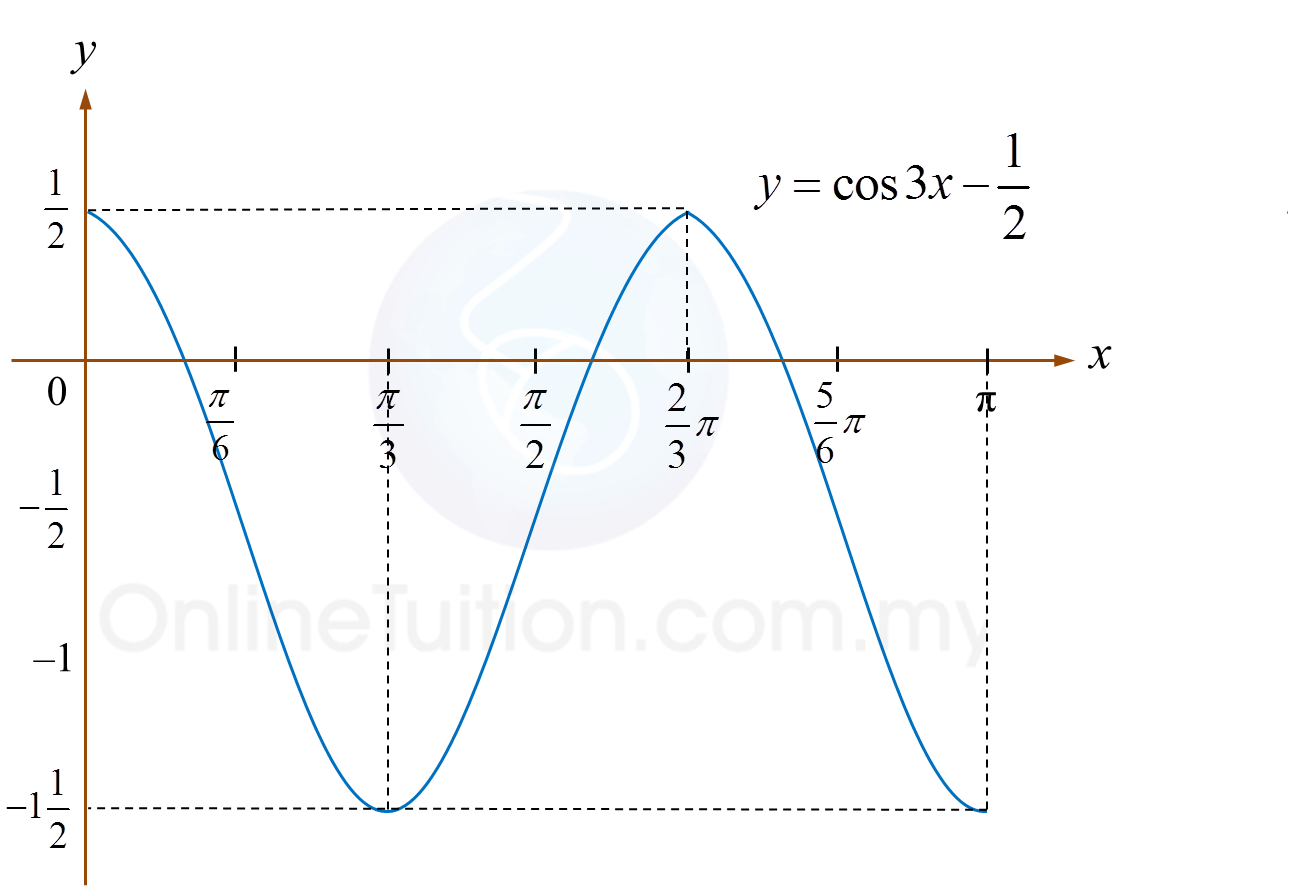
(b)(ii) y=sin(3x+π6)−sin(3x−π6)−12 for 0≤x≤π.y=cos3x−12
(a) Prove sin(3x+π6)−sin(3x−π6)=cos3x(b) Hence,(i) solve the equation sin(3x2+π6)−sin(3x2−π6)=12 for 0≤x≤2π and give your answer in the simplest fraction form in terms of π radian.(ii) sketch the graph of y=sin(3x+π6)−sin(3x−π6)−12 for 0≤x≤π.
Solution:
(a) Left hand side,sin(3x+π6)−sin(3x−π6)=[sin3xcosπ6+cos3xsinπ6]−[sin3xcosπ6−cos3xsinπ6]=2[cos3xsinπ6]=2[cos3x(12)]=cos3x(right hand side)
(b)(i)sin(3x2+π6)−sin(3x2−π6)=12,0≤x≤2πcos3x2=123x2=π3,(2π−π3),(2π+π3)3x2=π3,5π3,7π3x=2π9,10π9,14π9
(b)(ii) y=sin(3x+π6)−sin(3x−π6)−12 for 0≤x≤π.y=cos3x−12

Question 8 (10 marks):
Diagram 5 shows triangles OAQ and OPB where point P lies on OA and point Q lies on OB. The straight lines AQ and PB intersect at point R.
 It is given that →OA=18x˜, →OB=16y˜, OP:PA=1:2, OQ:QB=3:1,→PR=m→PB and →QR=n→QA, where m and n are constants.(a) Express →OR in terms of (i) m, x˜ and y˜, (ii) n, x˜ and y˜,(b) Hence, find the value of m and of n.(c) Given |x˜|=2 units, |y˜|=1 unit and OA is perpendicular to OB calculate |→PR|.
It is given that →OA=18x˜, →OB=16y˜, OP:PA=1:2, OQ:QB=3:1,→PR=m→PB and →QR=n→QA, where m and n are constants.(a) Express →OR in terms of (i) m, x˜ and y˜, (ii) n, x˜ and y˜,(b) Hence, find the value of m and of n.(c) Given |x˜|=2 units, |y˜|=1 unit and OA is perpendicular to OB calculate |→PR|.
Solution:
(a)(i)
→OR=→OP+→PR =13→OA+m→PB =13(18x˜)+m(→PO+→OB) =6x˜+m(−6x˜+16y˜)
(a)(ii)
→OR=→OQ+→QR =34→OB+n→QA =34(16y˜)+n(→QO+→OA) =12y˜+n(−12y˜+18x˜) =(12−12n)y˜+18nx˜
(b)
6x˜+m(−6x˜+16y˜)=(12−12n)y˜+18nx˜6x˜−6mx˜+16my˜=18nx˜+12y˜−12ny˜by comparison;6−6m=18n1−m=3nm=1−3n…………..(1)16m=12−12n4m=3−3n…………..(2)Substitute (1) into (2),4(1−3n)=3−3n4−12n=3−3n9n=1n=19Substitute n=19 into (1),m=1−3(19)m=23
(c)
|x˜|=2, |y˜|=1 →PR=23→PB =23(−6x˜+16y˜) =−4x˜+323y˜|→PR|=√[−4(2)]2+[323(1)]2 =√16009 =403 units
Diagram 5 shows triangles OAQ and OPB where point P lies on OA and point Q lies on OB. The straight lines AQ and PB intersect at point R.
 It is given that →OA=18x˜, →OB=16y˜, OP:PA=1:2, OQ:QB=3:1,→PR=m→PB and →QR=n→QA, where m and n are constants.(a) Express →OR in terms of (i) m, x˜ and y˜, (ii) n, x˜ and y˜,(b) Hence, find the value of m and of n.(c) Given |x˜|=2 units, |y˜|=1 unit and OA is perpendicular to OB calculate |→PR|.
It is given that →OA=18x˜, →OB=16y˜, OP:PA=1:2, OQ:QB=3:1,→PR=m→PB and →QR=n→QA, where m and n are constants.(a) Express →OR in terms of (i) m, x˜ and y˜, (ii) n, x˜ and y˜,(b) Hence, find the value of m and of n.(c) Given |x˜|=2 units, |y˜|=1 unit and OA is perpendicular to OB calculate |→PR|.
Solution:
(a)(i)
→OR=→OP+→PR =13→OA+m→PB =13(18x˜)+m(→PO+→OB) =6x˜+m(−6x˜+16y˜)
(a)(ii)
→OR=→OQ+→QR =34→OB+n→QA =34(16y˜)+n(→QO+→OA) =12y˜+n(−12y˜+18x˜) =(12−12n)y˜+18nx˜
(b)
6x˜+m(−6x˜+16y˜)=(12−12n)y˜+18nx˜6x˜−6mx˜+16my˜=18nx˜+12y˜−12ny˜by comparison;6−6m=18n1−m=3nm=1−3n…………..(1)16m=12−12n4m=3−3n…………..(2)Substitute (1) into (2),4(1−3n)=3−3n4−12n=3−3n9n=1n=19Substitute n=19 into (1),m=1−3(19)m=23
(c)
|x˜|=2, |y˜|=1 →PR=23→PB =23(−6x˜+16y˜) =−4x˜+323y˜|→PR|=√[−4(2)]2+[323(1)]2 =√16009 =403 units
Question 9 (10 marks):
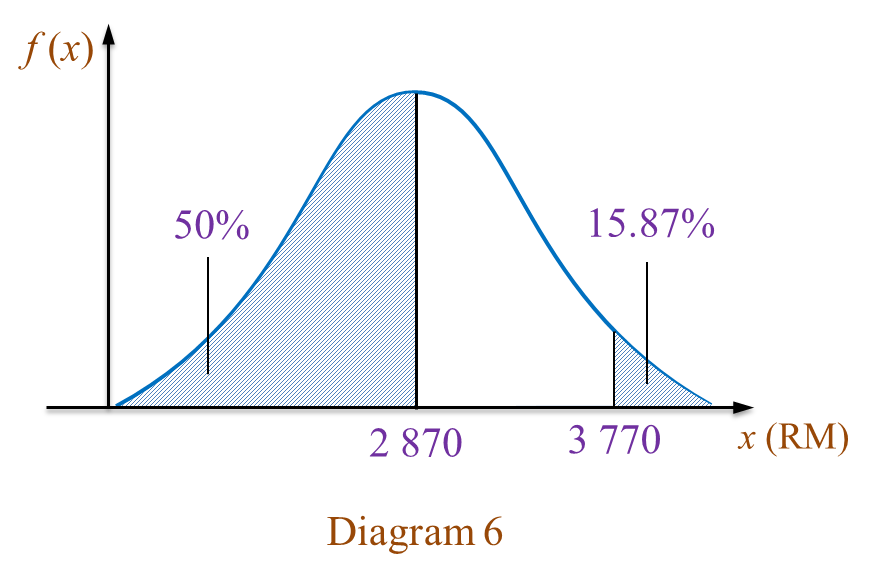
A study shows that the credit card balance of the customers is normally distributed as shown in Diagram 6.
 (a)(i) Find the standard deviation.
(a)(i) Find the standard deviation.
(ii) If 30 customers are chosen at random, find the number of customers who have a credit card balance between RM1800 and RM3000.
(b) It is found that 25% of the customers have a credit card balance less than RM y.
Find the value of y.
Solution:
(a)(i)
μ=2870,x=3770P(X>3770)=15.87%P(Z>3770−2870σ)=0.1587P(Z>1.0)=0.15873770−2870σ=1.0σ=900
(a)(ii)
P(1800<X<3000)=P(1800−2870900<Z<3000−2870900)=P(−1.189<Z<0.144)=1−P(Z≤−1.189)−P(Z≥0.144)=1−0.1172−0.4427=0.4401Number of customers=0.4401×30 =14
(b)
μ=2870,x=yP(x<y)=25%P(Z<y−2870900)=0.25y−2870900=−0.674y=2263.40
A study shows that the credit card balance of the customers is normally distributed as shown in Diagram 6.
 (a)(i) Find the standard deviation.
(a)(i) Find the standard deviation.(ii) If 30 customers are chosen at random, find the number of customers who have a credit card balance between RM1800 and RM3000.
(b) It is found that 25% of the customers have a credit card balance less than RM y.
Find the value of y.
Solution:
(a)(i)
μ=2870,x=3770P(X>3770)=15.87%P(Z>3770−2870σ)=0.1587P(Z>1.0)=0.15873770−2870σ=1.0σ=900
(a)(ii)
P(1800<X<3000)=P(1800−2870900<Z<3000−2870900)=P(−1.189<Z<0.144)=1−P(Z≤−1.189)−P(Z≥0.144)=1−0.1172−0.4427=0.4401Number of customers=0.4401×30 =14
(b)
μ=2870,x=yP(x<y)=25%P(Z<y−2870900)=0.25y−2870900=−0.674y=2263.40