Question 9:
Diagram 4 shows a circle ADBC, with centre O and diameter 12 cm while sector ADC with the centre A and radius AD = 10 cm.
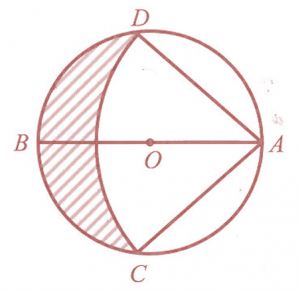 Diagram 4
Diagram 4
By using π = 3.142,
find
(a) ∠BAC in radians, (2 marks)
(b) area, in cm2, of the shaded region. (3 marks)
Solution:
(a)
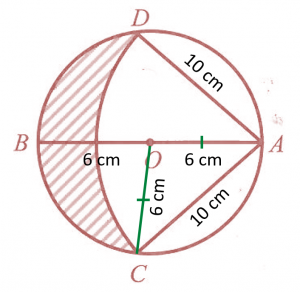
By using the cosine rule,62=102+62−2(10)(6)cos∠BACcos∠BAC=102+62−622(10)(6)cos∠BAC=0.8333∠BAC=33.56o×π180o =0.5857 rad
(b)
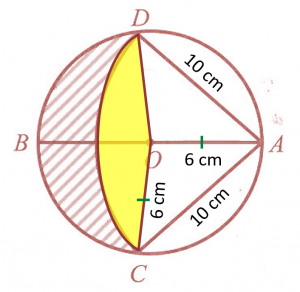
Area of sector CAD=12(10)2(0.5857×2) =58.57 cm2Area of yellow region=58.57−2(12(10)(6)sin33.56o)←12absinC=58.57−33.17=25.40 cm2∠COD=(0.5857×2)×2 =2.3428 radArea of shaded region=Area of sector CODB−Area of yellow region=12(6)2(2.3428)−25.40=16.77 cm2
Diagram 4 shows a circle ADBC, with centre O and diameter 12 cm while sector ADC with the centre A and radius AD = 10 cm.
 Diagram 4
Diagram 4By using π = 3.142,
find
(a) ∠BAC in radians, (2 marks)
(b) area, in cm2, of the shaded region. (3 marks)
Solution:
(a)

By using the cosine rule,62=102+62−2(10)(6)cos∠BACcos∠BAC=102+62−622(10)(6)cos∠BAC=0.8333∠BAC=33.56o×π180o =0.5857 rad
(b)

Area of sector CAD=12(10)2(0.5857×2) =58.57 cm2Area of yellow region=58.57−2(12(10)(6)sin33.56o)←12absinC=58.57−33.17=25.40 cm2∠COD=(0.5857×2)×2 =2.3428 radArea of shaded region=Area of sector CODB−Area of yellow region=12(6)2(2.3428)−25.40=16.77 cm2