Question 5:
Diagram below shows a triangle KLM.
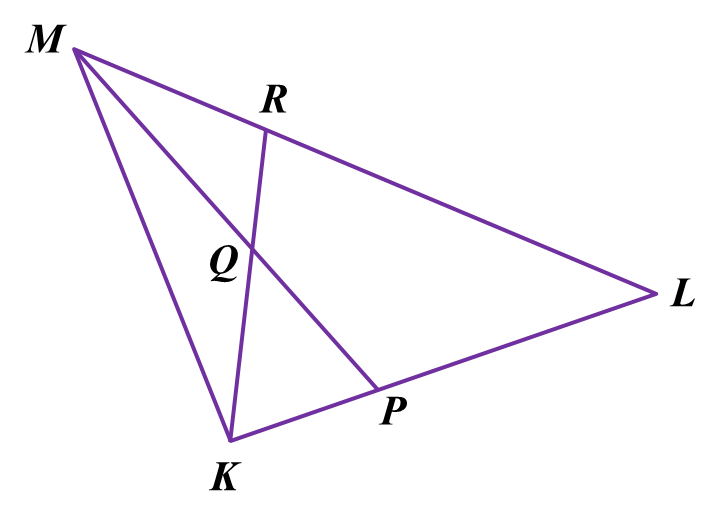
It is given that KP:PL=1:2, LR:RM=2:1, →KP=2x˜, →KM=3y˜.(a) Express in terms of x˜ and y˜,(i) →MP(ii) →MR(b) Given x˜=2i˜ and y˜=−i˜+4j˜, find →|MR|.(c) Given →MQ=h→MP and →QR=n→KR, where h and n are constants, find the value of h and of n.
Solution:
(a)(i)
→MP=→MK+→KP =−3y˜+2x˜ =2x˜−3y˜
(a)(ii)
→MR=13→ML =13(→MK+→KL) =13(−3y˜+6x˜) =2x˜−y˜
(b)
→MR=2(2i˜)−(−i˜+4j˜) =4i˜+i˜−4j˜ =5i˜−4j˜|→MR|=√52+(−4)2 =√41 units
(c)
→MQ+→QR=→MRh→MP+n→KR=→MRh(2x˜−3y˜)+n(→KM+→MR)=2x˜−y˜h(2x˜−3y˜)+n(3y˜+2x˜−y˜)=2x˜−y˜2hx˜−3hy˜+2nx˜+2ny˜=2x˜−y˜(2h+2n)x˜+(−3h+2n)y˜=2x˜−y˜2h+2n=2……….(1)−3h+2n=−1……….(2)(1)−(2):5h=3 h=35From (1):h+n=135+n=1 n=1−35 n=25
Diagram below shows a triangle KLM.

It is given that KP:PL=1:2, LR:RM=2:1, →KP=2x˜, →KM=3y˜.(a) Express in terms of x˜ and y˜,(i) →MP(ii) →MR(b) Given x˜=2i˜ and y˜=−i˜+4j˜, find →|MR|.(c) Given →MQ=h→MP and →QR=n→KR, where h and n are constants, find the value of h and of n.
Solution:
(a)(i)
→MP=→MK+→KP =−3y˜+2x˜ =2x˜−3y˜
(a)(ii)
→MR=13→ML =13(→MK+→KL) =13(−3y˜+6x˜) =2x˜−y˜
(b)
→MR=2(2i˜)−(−i˜+4j˜) =4i˜+i˜−4j˜ =5i˜−4j˜|→MR|=√52+(−4)2 =√41 units
(c)
→MQ+→QR=→MRh→MP+n→KR=→MRh(2x˜−3y˜)+n(→KM+→MR)=2x˜−y˜h(2x˜−3y˜)+n(3y˜+2x˜−y˜)=2x˜−y˜2hx˜−3hy˜+2nx˜+2ny˜=2x˜−y˜(2h+2n)x˜+(−3h+2n)y˜=2x˜−y˜2h+2n=2……….(1)−3h+2n=−1……….(2)(1)−(2):5h=3 h=35From (1):h+n=135+n=1 n=1−35 n=25
Question 6:
Diagram below shows a trapezium OABC and point D lies on AC.
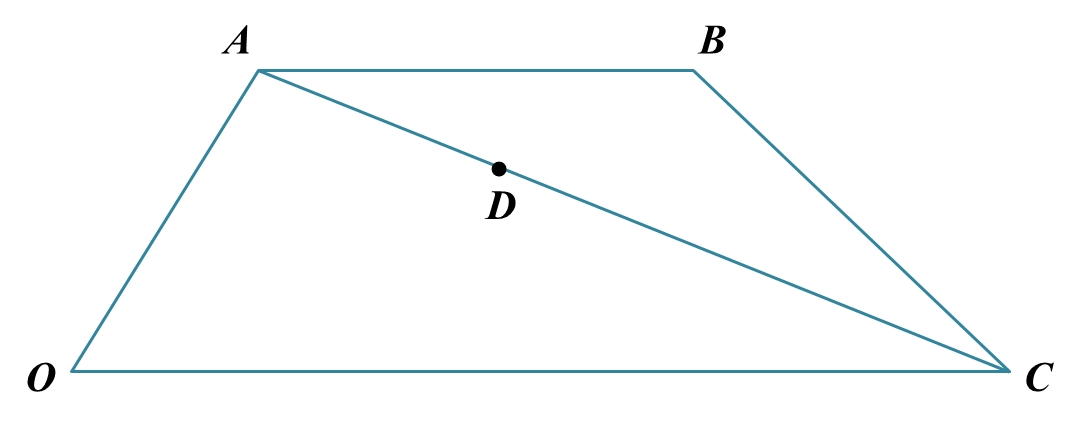
It is given that →OC=18b˜, →OA=6a˜ and →OC=2→AB.(a) Express in terms of a˜ and b˜,(i) →AC(ii) →OB(b) It is given that →AD=k→AC, where k is a constant.Find the value of k if the points O, D and B are collinear.
Solution:
(a)(i)
→AC=→AO+→OC =−6a˜+18b˜ =18b˜−6a˜
(a)(ii)
→OC=2→AB18b˜=2(→AO+→OB)18b˜=2(−6a˜+→OB)18b˜=−12a˜+2→OB→OB=6a˜+9b˜
(b)
→OD=h→OB=h(6a˜+9b˜)=6ha˜+9hb˜→AD=→OD−→OA=6ha˜+9hb˜−6a˜=a˜(6h−6)+9hb˜→AD=k→ACa˜(6h−6)+9hb˜=k(18b˜−6a˜)a˜(6h−6)+9hb˜=−6ka˜+18kb˜6h−6=−6kh−1=−kh=1−k……….(1)9h=18kh=2kFrom (1),1−k=2k3k=1k=13
Diagram below shows a trapezium OABC and point D lies on AC.

It is given that →OC=18b˜, →OA=6a˜ and →OC=2→AB.(a) Express in terms of a˜ and b˜,(i) →AC(ii) →OB(b) It is given that →AD=k→AC, where k is a constant.Find the value of k if the points O, D and B are collinear.
Solution:
(a)(i)
→AC=→AO+→OC =−6a˜+18b˜ =18b˜−6a˜
(a)(ii)
→OC=2→AB18b˜=2(→AO+→OB)18b˜=2(−6a˜+→OB)18b˜=−12a˜+2→OB→OB=6a˜+9b˜
(b)
→OD=h→OB=h(6a˜+9b˜)=6ha˜+9hb˜→AD=→OD−→OA=6ha˜+9hb˜−6a˜=a˜(6h−6)+9hb˜→AD=k→ACa˜(6h−6)+9hb˜=k(18b˜−6a˜)a˜(6h−6)+9hb˜=−6ka˜+18kb˜6h−6=−6kh−1=−kh=1−k……….(1)9h=18kh=2kFrom (1),1−k=2k3k=1k=13