Question 3:
Solution:
(a)(i)
→QS=→QP+→PS→QS=−20x˜+32y˜←Given →PT=14→PS→PS=4→PT=4(8y˜)=32y˜
(a)(ii)
→TR=→TS+→SR→TR=34→PS+25x˜−24y˜→TR=34(32y˜)+25x˜−24y˜→TR=24y˜+25x˜−24y˜→TR=25x˜
(b)
→QU=→QP+→PT+→TU→QU=−20x˜+8y˜+35(25x˜)←Given→TU=35→TR→QU=−20x˜+8y˜+15x˜→QU=−5x˜+8y˜From (a)(i) →QS=−20x˜+32y˜→QS→QU=−20x˜+32y˜−5x˜+8y˜→QS→QU=4(−5x˜+8y˜)(−5x˜+8y˜)→QS→QU=4→QS=4→QU∴ Q, U and S are collinear.
(c)
→PS=32y˜|→PS|=32|y˜||→PS|=32×3=96→PQ=20x˜|→PQ|=20|x˜||→PQ|=20×2=40∴|→QS|=√962+402|→QS|=104
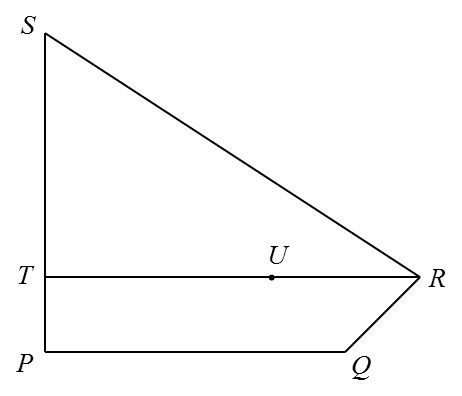
In diagram below, PQRS is a quadrilateral. PTS and TUR are straight lines.
It is given that
→PQ=20x˜, →PT=8y˜, →SR=25x˜−24y˜, →PT=14→PS and →TU=35→TR
(a) Express in terms of
x˜
and/or
y˜
:
(i)
→QS
(ii) →TR
(b) Show that the points Q, U and S are collinear.
(ii) →TR
(b) Show that the points Q, U and S are collinear.
(c) If
|x˜|
= 2 and
|y˜|
= 3, find
|→QS|
Solution:
(a)(i)
→QS=→QP+→PS→QS=−20x˜+32y˜←Given →PT=14→PS→PS=4→PT=4(8y˜)=32y˜
(a)(ii)
→TR=→TS+→SR→TR=34→PS+25x˜−24y˜→TR=34(32y˜)+25x˜−24y˜→TR=24y˜+25x˜−24y˜→TR=25x˜
(b)
→QU=→QP+→PT+→TU→QU=−20x˜+8y˜+35(25x˜)←Given→TU=35→TR→QU=−20x˜+8y˜+15x˜→QU=−5x˜+8y˜From (a)(i) →QS=−20x˜+32y˜→QS→QU=−20x˜+32y˜−5x˜+8y˜→QS→QU=4(−5x˜+8y˜)(−5x˜+8y˜)→QS→QU=4→QS=4→QU∴ Q, U and S are collinear.
(c)
→PS=32y˜|→PS|=32|y˜||→PS|=32×3=96→PQ=20x˜|→PQ|=20|x˜||→PQ|=20×2=40∴|→QS|=√962+402|→QS|=104
Question 4:
Diagram below shows quadrilateral ABCD. The straight line AC intersects the straight line BD at point E.
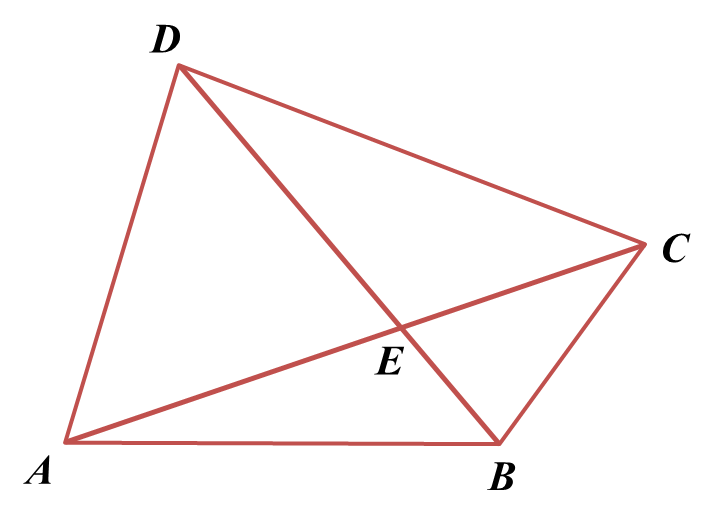
It is given that BE:ED=2:3, →AB=10x˜, →AD=25y˜ and →BC=−x˜+15y˜.(a) Express in terms of x˜ and y˜,(i) →BD(ii) →AE(b) Find the ratio AE:EC.
Solution:
(a)(i)
→BD=→BA+→AD =→AD−→AB =25y˜−10x˜
(a)(ii)
→AE=→AB+→BE =→AB+25→BD =10x˜+25(25y˜−10x˜) =10x˜+25(25y˜−10x˜) =10x˜+10y˜−4x˜ =6x˜+10y˜ =2(3x˜+5y˜)
(b)
→EC=→EB+→BC =→BC−→BE =→BC−23→ED =→BC−23(→EA+→AD) =−x˜+15y˜−23(−6x˜−10y˜+25y˜) =−x˜+15y˜−23(−6x˜+15y˜) =−x˜+15y˜+4x˜−10y˜ =3x˜+5y˜AEEC=2(3x˜+5y˜)1(3x˜+5y˜)AE:EC=2:1
Diagram below shows quadrilateral ABCD. The straight line AC intersects the straight line BD at point E.

It is given that BE:ED=2:3, →AB=10x˜, →AD=25y˜ and →BC=−x˜+15y˜.(a) Express in terms of x˜ and y˜,(i) →BD(ii) →AE(b) Find the ratio AE:EC.
Solution:
(a)(i)
→BD=→BA+→AD =→AD−→AB =25y˜−10x˜
(a)(ii)
→AE=→AB+→BE =→AB+25→BD =10x˜+25(25y˜−10x˜) =10x˜+25(25y˜−10x˜) =10x˜+10y˜−4x˜ =6x˜+10y˜ =2(3x˜+5y˜)
(b)
→EC=→EB+→BC =→BC−→BE =→BC−23→ED =→BC−23(→EA+→AD) =−x˜+15y˜−23(−6x˜−10y˜+25y˜) =−x˜+15y˜−23(−6x˜+15y˜) =−x˜+15y˜+4x˜−10y˜ =3x˜+5y˜AEEC=2(3x˜+5y˜)1(3x˜+5y˜)AE:EC=2:1