SPM Additional Mathematics 2018, Paper 1 (Question 14 – 16)

Question 14 (4 marks):
It is given that p, 2 and q are the first three terms of a geometric progression.
Express in terms of q
(a) the first term and the common ratio of the progression.
(b) the sum to infinity of the progression.

Solution:
(a)
$\begin{array}{l}{T}_1=p,T_2=2,T_3=q\\ \frac{T_2}{T_1}=\frac{T_3}{T_2}\\ \frac{2}{p}=\frac{q}{2}\\ p=\frac{4}{q}\\ \mathrm{First}\text{ term},T_1=p=\frac{4}{q}\\ \text{Common ratio}=\frac{q}{2}\end{array}$

(b)
$\begin{array}{l}a=\frac{4}{q},r=\frac{q}{2}\\ S_{\infty }=\frac{a}{1-r}\\ =\frac{\frac{4}{q}}{1-\frac{q}{2}}\\ =\frac{4}{q}÷\left[1-\frac{q}{2}\right]\\ =\frac{4}{q}÷\left[\frac{2-q}{2}\right]\\ =\frac{4}{q}\times \frac{2}{2-q}\\ =\frac{8}{2q-q^2}\end{array}$

Question 15 (3 marks):
A student has a wire with the length of 13.16 m. The student divided the wire into several pieces. Each piece is to form a square. Diagram 7 shows the first three squares formed by the student.


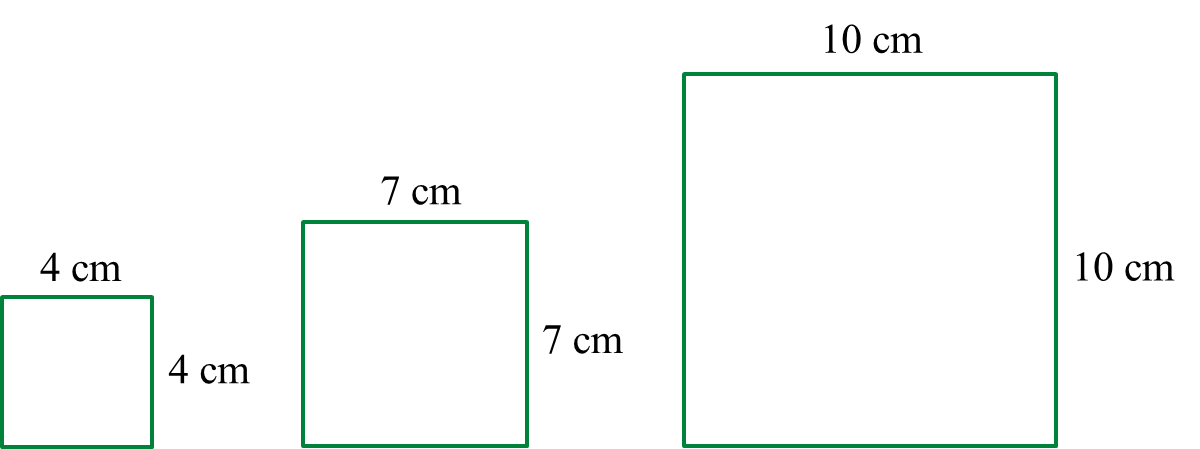
Diagram 7

How many squares can be formed by the student?


Solution:
$\begin{array}{l}\text{Perimeter of squares;}\\ T_1=4\left(4\right)=16\text{ cm}\\ T_2=4\left(7\right)=28\text{ cm}\\ T_3=4\left(10\right)=40\text{ cm}\\ \\ \text{First term, }a=16,\\ \text{Common difference, }d\\ =28-16\\ =12\\ \\ \text{Total perimeter, }{S}_n=13.16\text{ m}=1316\text{ cm}\\ \frac{n}{2}\left[2\left(16\right)+\left(n-1\right)12\right]=1316\\ n\left[32+12n-12\right]=2632\\ 12n^2+20n-2632=0\\ 3n^2+5n-658=0\\ \left(n-14\right)\left(3n+47\right)=0\\ n-14=0\\ n=14\\ \text{Or}\\ 3n+47=0\\ n=-\frac{47}{3}\left(\text{rejected}\right)\\ \\ 14\text{ squares can be formed using 13}\text{.16 m of wire}\text{.}\end{array}$

Question 16 (2 marks):
Given 2^p + 2^p = 2^k. Express p in terms of k.

Solution:
$\begin{array}{l}{2}^p+2^p=2^k\\ 2\left(2^p\right)=2^k\\ 2^p=\frac{2^k}{2^1}\\ 2^p=2^{k-1}\\ p=k-1\end{array}$