Question 12 (4 marks):
Diagram 5 shows a circle with centre O.
 Diagram 5
Diagram 5
PR and QR are tangents to the circle at points P and Q respectively. It is given that the length of minor arc PQ is 4 cm and OR=5α cm.
Express in terms of α,
(a) the radius, r, of the circle,
(b) the area, A, of the shaded region.
Solution:
(a)
Given sPQ=4 rα=4 r=4α cm
(b)
PR=√(5α)2−(4α)2PR=√9α2PR=3αA= Area of shaded regionA= Area of quadrilateral OPRQ−Area of sector OPQ=2(Area of △ OPR)−12r2θ=2[12×3α×4α]−[12×(4α)2×α]=12α2−8α=12−8αα2 cm2
Diagram 5 shows a circle with centre O.
 Diagram 5
Diagram 5PR and QR are tangents to the circle at points P and Q respectively. It is given that the length of minor arc PQ is 4 cm and OR=5α cm.
Express in terms of α,
(a) the radius, r, of the circle,
(b) the area, A, of the shaded region.
Solution:
(a)
Given sPQ=4 rα=4 r=4α cm
(b)
PR=√(5α)2−(4α)2PR=√9α2PR=3αA= Area of shaded regionA= Area of quadrilateral OPRQ−Area of sector OPQ=2(Area of △ OPR)−12r2θ=2[12×3α×4α]−[12×(4α)2×α]=12α2−8α=12−8αα2 cm2
Question 13 (3 marks):
Diagram 6 shows the graph of a straight line x2y against 1x.
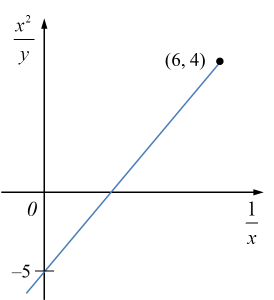 Diagram 6
Diagram 6
Based on Diagram 6, express y in terms of x.
Solution:
m=4−(−5)6−0=32c=−5Y=x2y, X=1xY=mX+cx2y=32(1x)+(−5)x2y=32x−5x2y=3−10x2xyx2=2x3−10xy=2x33−10x
Diagram 6 shows the graph of a straight line x2y against 1x.
 Diagram 6
Diagram 6Based on Diagram 6, express y in terms of x.
Solution:
m=4−(−5)6−0=32c=−5Y=x2y, X=1xY=mX+cx2y=32(1x)+(−5)x2y=32x−5x2y=3−10x2xyx2=2x3−10xy=2x33−10x