Question 8 (3 marks):
Diagram 3 shows vectors →OP, →OQ and →OM drawn on a square grid.
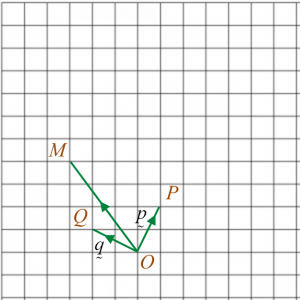 Diagram 3
Diagram 3
(a) Express →OM in the form hp˜+kq˜, where h and k are constants.(b) On the diagram 3, mark and label the point N such that →MN+→OQ=2→OP.
Solution:
(a)
→OM=p˜+2q˜
(b)
→MN+→OQ=2→OP→MN=2→OP−→OQ =2p˜−q˜
Diagram 3 shows vectors →OP, →OQ and →OM drawn on a square grid.
 Diagram 3
Diagram 3(a) Express →OM in the form hp˜+kq˜, where h and k are constants.(b) On the diagram 3, mark and label the point N such that →MN+→OQ=2→OP.
Solution:
(a)
→OM=p˜+2q˜
(b)
→MN+→OQ=2→OP→MN=2→OP−→OQ =2p˜−q˜
Question 9 (4 marks):
A(2, 3) and B(−2, 5) lie on a Cartesian plane.It is given that 3→OA=2→OB+→OC.Find(a) the coordinates of C,(b) |→AC|
Solution:
Given A(2,3) and B(−2,5)Thus, →OA=2i˜+3j˜ and →OB=−2i˜+5j˜
(a)
3→OA=2→OB+→OC→OC=3→OA−2→OB =3(2i˜+3j˜)−2(−2i˜+5j˜) =6i˜+9j˜+4i˜−10j˜ =10i˜−j˜Thus, coordinate of C is (10, −1)
(b)
→AC=→AO+→OC =−→OA+→OC =−(2i˜+3j˜)+10i˜−j˜ =−2i˜+10i˜−3j˜−j˜ =8i˜−4j˜|→AC|=√82+(−4)2 =√80 units =√16×5 units =4√5 units
A(2, 3) and B(−2, 5) lie on a Cartesian plane.It is given that 3→OA=2→OB+→OC.Find(a) the coordinates of C,(b) |→AC|
Solution:
Given A(2,3) and B(−2,5)Thus, →OA=2i˜+3j˜ and →OB=−2i˜+5j˜
(a)
3→OA=2→OB+→OC→OC=3→OA−2→OB =3(2i˜+3j˜)−2(−2i˜+5j˜) =6i˜+9j˜+4i˜−10j˜ =10i˜−j˜Thus, coordinate of C is (10, −1)
(b)
→AC=→AO+→OC =−→OA+→OC =−(2i˜+3j˜)+10i˜−j˜ =−2i˜+10i˜−3j˜−j˜ =8i˜−4j˜|→AC|=√82+(−4)2 =√80 units =√16×5 units =4√5 units
Question 10 (3 marks):
The following information refers to the equation of two straight lines, AB and CD.
AB:y−2kx−3=0 CD:x3h+y4=1where h and k are constants.
Given the straight lines AB and CD are perpendicular to each other, express h in terms of k.
Solution:
AB:y−2kx−3=0y=2kx+3mAB=2kCD:x3h+y4=1mCD=−43hmAB×mCD=−12k×(−43h)=−1−8k=−3hh=83k
The following information refers to the equation of two straight lines, AB and CD.
AB:y−2kx−3=0 CD:x3h+y4=1where h and k are constants.
Given the straight lines AB and CD are perpendicular to each other, express h in terms of k.
Solution:
AB:y−2kx−3=0y=2kx+3mAB=2kCD:x3h+y4=1mCD=−43hmAB×mCD=−12k×(−43h)=−1−8k=−3hh=83k