Question 7:
Diagram below shows quadrilateral OPBC. The straight line AC intersects the straight line PQ at point B.
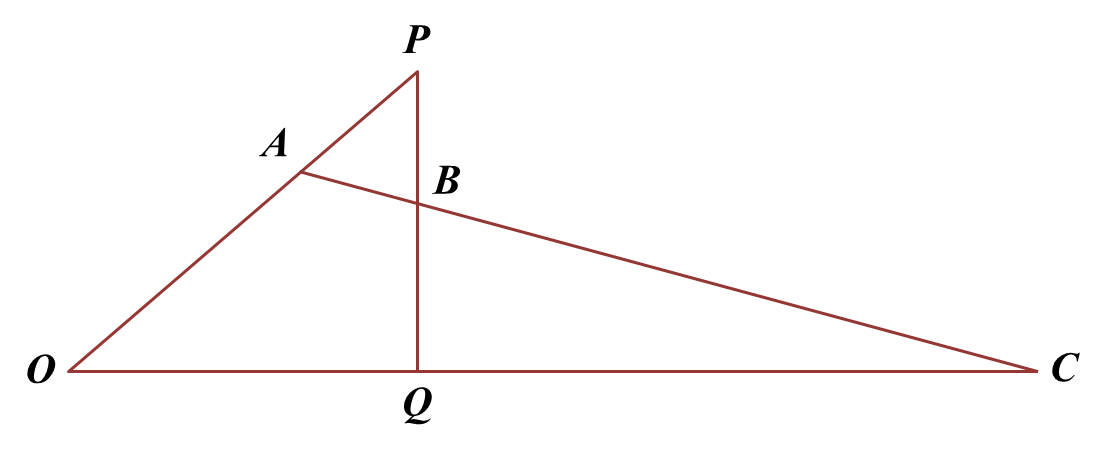
It is given that →OP=a˜, →OQ=b˜, →OA=4→AP, →OC=3→OQ, →PB=h→PQ and→AB=k→AC.(a) Express →OB in terms of h, a˜ and b˜.(b) Express →OB in terms of k, a˜ and b˜.(c)(i) Find the value of h and of k.(ii) Hence, state →OB in terms of a˜ and b˜.
Solution:
(a)
→OB=→OP+→PB =a˜+h→PQ =a˜+h(→PO+→OQ) =a˜+h(−a˜+b˜) =a˜−ha˜+hb˜→OB=(1−h)a˜+hb˜
(b)
→OB=→OP+→PB =a˜+→PA+→AB =a˜+(−15→OP)+k→AC =a˜+(−15a˜)+k(→AO+→OC) =45a˜+k(−45→OP+3→OQ) =45a˜+k(−45a˜+3b˜) =45a˜−45ka˜+3kb˜→OB=45(1−k)a˜+3kb˜
(c)(i)
(1−h)a˜+hb˜=45(1−k)a˜+3kb˜1−h=45−45k……….(1)h=3k……….(2)Substitute (2) into the (1) 1−3k=45−45k5−15k=4−4k11k=1k=111Substitute k=111 into (2)h=3(111) =311
(c)(ii)
→OB=(1−h)a˜+hb˜when h=311=(1−311)a˜+(311)b˜=811a˜+311b˜
Diagram below shows quadrilateral OPBC. The straight line AC intersects the straight line PQ at point B.

It is given that →OP=a˜, →OQ=b˜, →OA=4→AP, →OC=3→OQ, →PB=h→PQ and→AB=k→AC.(a) Express →OB in terms of h, a˜ and b˜.(b) Express →OB in terms of k, a˜ and b˜.(c)(i) Find the value of h and of k.(ii) Hence, state →OB in terms of a˜ and b˜.
Solution:
(a)
→OB=→OP+→PB =a˜+h→PQ =a˜+h(→PO+→OQ) =a˜+h(−a˜+b˜) =a˜−ha˜+hb˜→OB=(1−h)a˜+hb˜
(b)
→OB=→OP+→PB =a˜+→PA+→AB =a˜+(−15→OP)+k→AC =a˜+(−15a˜)+k(→AO+→OC) =45a˜+k(−45→OP+3→OQ) =45a˜+k(−45a˜+3b˜) =45a˜−45ka˜+3kb˜→OB=45(1−k)a˜+3kb˜
(c)(i)
(1−h)a˜+hb˜=45(1−k)a˜+3kb˜1−h=45−45k……….(1)h=3k……….(2)Substitute (2) into the (1) 1−3k=45−45k5−15k=4−4k11k=1k=111Substitute k=111 into (2)h=3(111) =311
(c)(ii)
→OB=(1−h)a˜+hb˜when h=311=(1−311)a˜+(311)b˜=811a˜+311b˜
Question 8:
Diagram below shows quadrilateral OPQR. The straight line PR intersects the straight line OQ at point S.

It is given that →OP=7x˜, →OR=5y˜, PS:SR=3:1 and →OR is parallel to →PQ.(a) Express in terms of x˜ and y˜,(i) →PR(ii) →OS(b) Using →PQ=m→OR and →SQ=n→OS, where m and n are constants, Find the value of m and of n.(c) Given that |y˜|=4 units and the area of ORS is 50 cm2, find the perpendicular distance from point S to OR.
Solution:
(a)(i)
→PR=→PO+→OR =−7x˜+5y˜
(a)(ii)
→OS=→OP+→PS =7x˜+34→PR =7x˜+34(−7x˜+5y˜) =7x˜−214x˜+154y˜ =74x˜+154y˜
(b)
→PS=→PQ−→SQ34→PR=m→OR−n→OS34(−7x˜+5y˜)=m(5y˜)−n(74x˜+154y˜)−214x˜+154y˜=5my˜−74nx˜−154ny˜−214x˜+154y˜=−74nx˜+5my˜−154ny˜−74n=−2147n=21n=35m−154n=1545m−154(3)=1545m−454=1545m=15m=3
(c)
Area of ΔORS=5012×(5y˜)×t=5012×5(4)×t=5010t=50t=5∴ Perpendicular distance from point S to OR=5 units.
Diagram below shows quadrilateral OPQR. The straight line PR intersects the straight line OQ at point S.

It is given that →OP=7x˜, →OR=5y˜, PS:SR=3:1 and →OR is parallel to →PQ.(a) Express in terms of x˜ and y˜,(i) →PR(ii) →OS(b) Using →PQ=m→OR and →SQ=n→OS, where m and n are constants, Find the value of m and of n.(c) Given that |y˜|=4 units and the area of ORS is 50 cm2, find the perpendicular distance from point S to OR.
Solution:
(a)(i)
→PR=→PO+→OR =−7x˜+5y˜
(a)(ii)
→OS=→OP+→PS =7x˜+34→PR =7x˜+34(−7x˜+5y˜) =7x˜−214x˜+154y˜ =74x˜+154y˜
(b)
→PS=→PQ−→SQ34→PR=m→OR−n→OS34(−7x˜+5y˜)=m(5y˜)−n(74x˜+154y˜)−214x˜+154y˜=5my˜−74nx˜−154ny˜−214x˜+154y˜=−74nx˜+5my˜−154ny˜−74n=−2147n=21n=35m−154n=1545m−154(3)=1545m−454=1545m=15m=3
(c)
Area of ΔORS=5012×(5y˜)×t=5012×5(4)×t=5010t=50t=5∴ Perpendicular distance from point S to OR=5 units.