Question 1:
Diagram below shows the relation between set M and set N in the graph form.
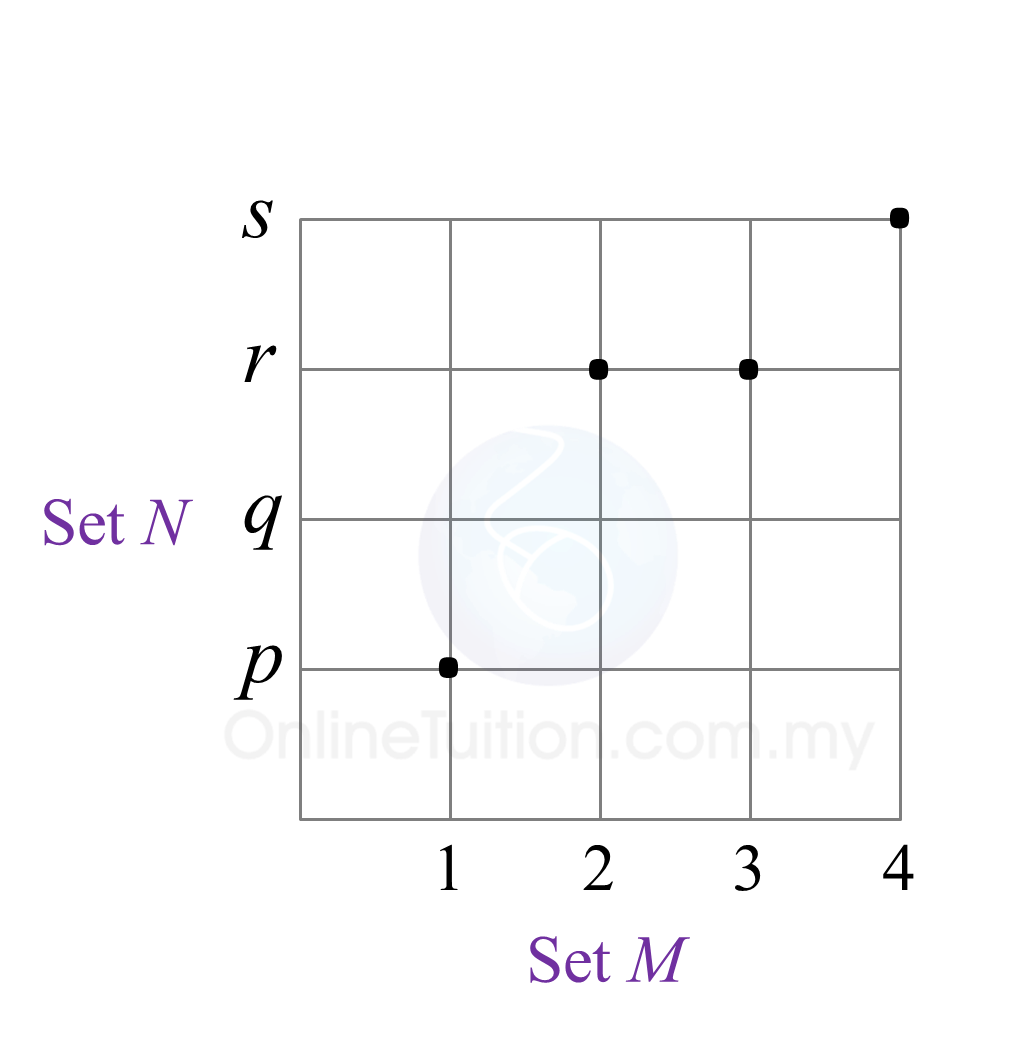 State
State
(a) the range of the relation,
(b) the type of the relation between set M and set N.
Solution:
(a) Range of the relation = {p, r, s}.
(b) Type of the relation between set M and set N is many to one relation.
Diagram below shows the relation between set M and set N in the graph form.
 State
State(a) the range of the relation,
(b) the type of the relation between set M and set N.
Solution:
(a) Range of the relation = {p, r, s}.
(b) Type of the relation between set M and set N is many to one relation.
Question 2:
Diagram below shows the relation between set P and set Q.
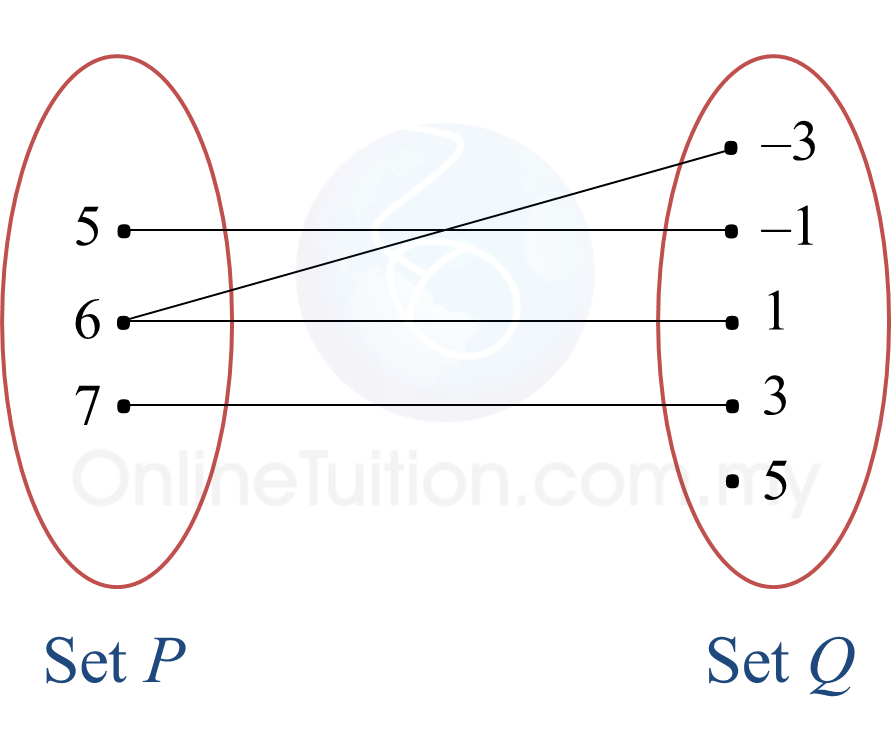
State
(a) the object of 3,
(b) the range of the relation.
Solution:
(a) The object of 3 is 7.
(b) The range of the relation is {–3, –1, 1, 3}.
Diagram below shows the relation between set P and set Q.

State
(a) the object of 3,
(b) the range of the relation.
Solution:
(a) The object of 3 is 7.
(b) The range of the relation is {–3, –1, 1, 3}.
Question 3:
Diagram below shows the function g : x → x – 2k, where k is a constant.
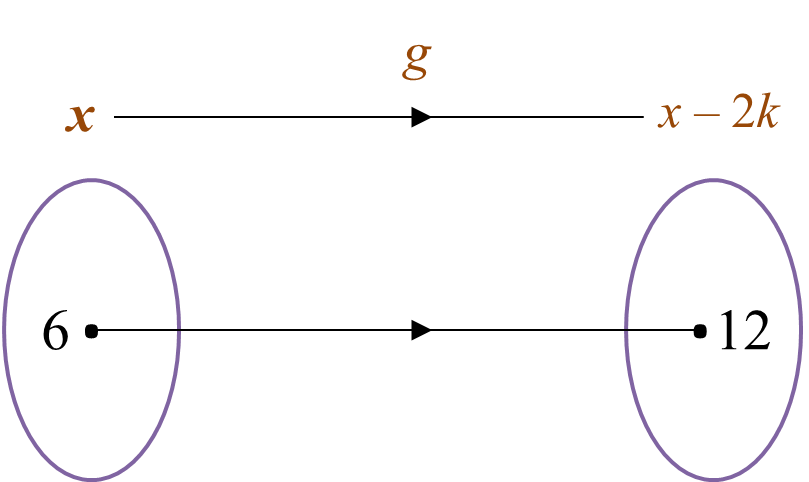
Find the value of k.
Solution:
Given g(6)=12 g(6)=6−2k 12=6−2k 2k=6−12 k=−3
Diagram below shows the function g : x → x – 2k, where k is a constant.

Find the value of k.
Solution:
Given g(6)=12 g(6)=6−2k 12=6−2k 2k=6−12 k=−3
Question 4:
Diagram below shows the relation between set M and set N in the arrow diagram.

(a) Represent the relation in the form of ordered pairs.
(b) State the domain of the relation.
Solution:
(a) Relation in the form of ordered pairs = {(–4, 8), (3, 3), (4, 8)}.
(b) Domain of the relation = {–4, 3, 4}.
Diagram below shows the relation between set M and set N in the arrow diagram.

(a) Represent the relation in the form of ordered pairs.
(b) State the domain of the relation.
Solution:
(a) Relation in the form of ordered pairs = {(–4, 8), (3, 3), (4, 8)}.
(b) Domain of the relation = {–4, 3, 4}.