5.3.3 Arithmetic Progressions, SPM Practice (Paper 1)

Question 11:
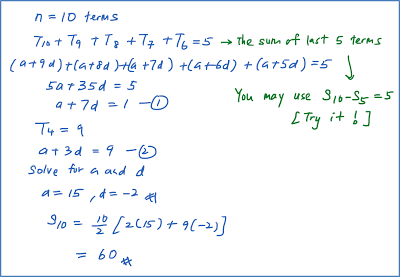
An arithmetic progression consists of 10 terms. The sum of the last 5 terms is 5 and the fourth term is 9. Find the sum of this progression.

Solution:

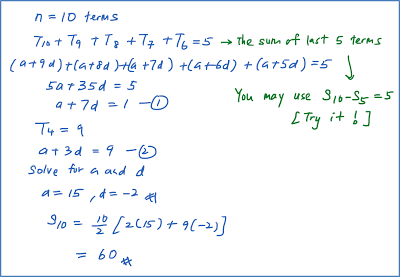
Question 12:
The sum of the first 6 terms of an arithmetic progression is 39 and the sum of the next 6 terms is –69. Find
(a) The first term and the common difference.
(b) The sum of all the terms from the 15th term to the 25th term.

Solution:

Question 13:
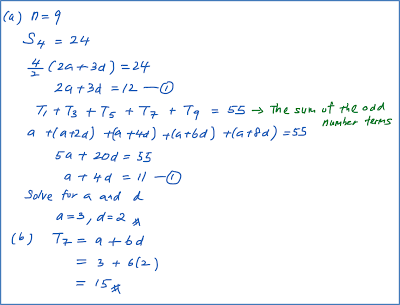
An arithmetic progression has 9 terms. The sum of the first four terms is 24 and the sum of all the odd number terms is 55. Find
(a) The first term and common difference,
(b) The seventh term.

Solution:

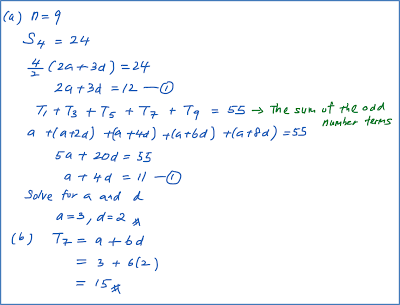
Question 14:
An arithmetic series, with the first term 12 and common difference d, consists of 23 terms. Given that the sum of the last 3 terms is 5 times the sum of the first 3 terms, find
(a) the value of d,
(b) the sum of the first 19 terms.

Solution:

Question 15:
The sum of n terms of an arithmetic progression is given by the formula $S_n=\frac{n}{2}(5-3n)$ . Find
(a) the first term,
(b) the common difference,
(c) the tenth term.

Solution: