\

2.6 Gradients of Tangents, Equations of Tangents and Normals

2.6 Gradients of Tangents, Equations of Tangents and Normals



If A(x1, y1) is a point on a line y = f(x), the gradient of the line (for a straight line) or the gradient of the tangent of the line (for a curve) is the value of d y d x when x = x1.

(A) Gradient of tangent at A(x1, y1):




(B) Equation of tangent:




(C) Gradient of normal at A(x1, y1):






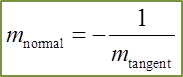
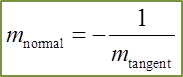
(D) Equation of normal :  




Example 1 (Find the Equation of Tangent)
Given that y = 4 ( 3 x − 1 ) 2 . Find the equation of the tangent at the point (1, 1).

Solution:
y = 4 ( 3 x − 1 ) 2 = 4 ( 3 x − 1 ) − 2 d y d x = − 2.4 ( 3 x − 1 ) − 3 .3 d y d x = − 24 ( 3 x − 1 ) 3 At point  ( 1 ,  1 ) ,   d y d x = − 24 [ 3 ( 1 ) − 1 ] 3 = − 24 8 = − 3 Equation of tangent at point  ( 1 ,  1 )  is, y − 1 = − 3 ( x − 1 ) y − 1 = − 3 x + 3 y = − 3 x + 4


Example 2 (Find the Equation of Normal)
Find the gradient of the curve y = 7 3 x + 4 at the point (-1, 7). Hence, find the equation of the normal to the curve at this point.

Solution:
y = 7 3 x + 4 = 7 ( 3 x + 4 ) − 1 d y d x = − 7 ( 3 x + 4 ) − 2 .3 d y d x = − 21 ( 3 x + 4 ) 2 At point  ( − 1 ,   7 ) ,   d y d x = − 21 [ 3 ( − 1 ) + 4 ] 2 = − 21 Gradient of the normal  = 1 21 Equation of the normal is y − y 1 = m ( x − x 1 ) y − 7 = 1 21 ( x − ( − 1 ) ) 21 y − 147 = x + 1 21 y − x − 148 = 0

Leave a Comment