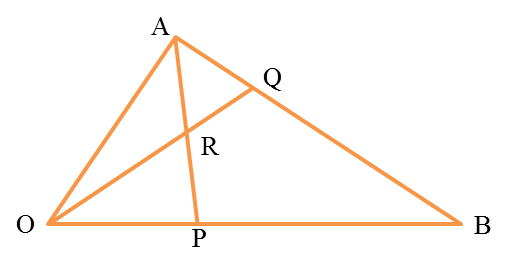
Question 1:
The above diagram shows triangle OAB. The straight line AP intersects the straight line OQ at R. It is given that OP=14OB, AQ=14AB, →OP=4b˜ and →OA=8a˜.OP=14OB, AQ=14AB, −−→OP=4b˜ and −−→OA=8a˜.
(a) Express in terms of a˜ and/ or b˜:a˜ and/ or b˜:
(i)→AP(ii)→OQ(i)−−→AP(ii)−−→OQ
(b)(i) Given that →AR=h→AP−−→AR=h−−→AP , state →AR−−→AR in terms of h, a˜ and b˜.a˜ and b˜.
(ii) Given that →RQ=k→OQ,−−→RQ=k−−→OQ, state in terms of k, a˜ and b˜.a˜ and b˜.
(c) Using →AQ=→AR+→RQ,−−→AQ=−−→AR+−−→RQ, find the value of h and of k.
Solution:
(a)(i)
→AP=→AO+→OP→AP=−→OA+→OP→AP=−8a˜+4b˜−−→AP=−−→AO+−−→OP−−→AP=−−−→OA+−−→OP−−→AP=−8a˜+4b˜
(a)(ii)
→OQ=→OA+→AQ→OQ=8a˜+14→AB→OQ=8a˜+14(→AO+→OB)→OQ=8a˜+14(−8a˜+4→OP)→OQ=8a˜+14(−8a˜+4(4b˜))→OQ=8a˜−2a˜+4b˜→OQ=6a˜+4b˜−−→OQ=−−→OA+−−→AQ−−→OQ=8a˜+14−−→AB−−→OQ=8a˜+14(−−→AO+−−→OB)−−→OQ=8a˜+14(−8a˜+4−−→OP)−−→OQ=8a˜+14(−8a˜+4(4b˜))−−→OQ=8a˜−2a˜+4b˜−−→OQ=6a˜+4b˜
(b)(i)
→AR=h→AP→AR=h(−8a˜+4b˜)→AR=−8ha˜+4hb˜−−→AR=h−−→AP−−→AR=h(−8a˜+4b˜)−−→AR=−8ha˜+4hb˜
(b)(ii)
→RQ=k→OQ→RQ=k(6a˜+4b˜)→RQ=6ka˜+4kb˜−−→RQ=k−−→OQ−−→RQ=k(6a˜+4b˜)−−→RQ=6ka˜+4kb˜
(c)
→AQ=→AR+→RQ→AQ=−8ha˜+4hb˜+(6ka˜+4kb˜)→AO+→OQ=−8ha˜+4hb˜+6ka˜+4kb˜−8a˜+6a˜+4b˜=−8ha˜+6ka˜+4hb˜+4kb˜−2a˜+4b˜=−8ha˜+6ka˜+4hb˜+4kb˜−2=−8h+6k−1=−4h+3k→(1)4=4h+4k1=h+kk=1−h→(2)Substitute (2) into (1),−1=−4h+3(1−h)−1=−4h+3−3h−4=−7hh=47From (2),k=1−47=37−−→AQ=−−→AR+−−→RQ−−→AQ=−8ha˜+4hb˜+(6ka˜+4kb˜)−−→AO+−−→OQ=−8ha˜+4hb˜+6ka˜+4kb˜−8a˜+6a˜+4b˜=−8ha˜+6ka˜+4hb˜+4kb˜−2a˜+4b˜=−8ha˜+6ka˜+4hb˜+4kb˜−2=−8h+6k−1=−4h+3k→(1)4=4h+4k1=h+kk=1−h→(2)Substitute (2) into (1),−1=−4h+3(1−h)−1=−4h+3−3h−4=−7hh=47From (2),k=1−47=37
The above diagram shows triangle OAB. The straight line AP intersects the straight line OQ at R. It is given that OP=14OB, AQ=14AB, →OP=4b˜ and →OA=8a˜.OP=14OB, AQ=14AB, −−→OP=4b˜ and −−→OA=8a˜.
(a) Express in terms of a˜ and/ or b˜:a˜ and/ or b˜:
(i)→AP(ii)→OQ(i)−−→AP(ii)−−→OQ
(b)(i) Given that →AR=h→AP−−→AR=h−−→AP , state →AR−−→AR in terms of h, a˜ and b˜.a˜ and b˜.
(ii) Given that →RQ=k→OQ,−−→RQ=k−−→OQ, state in terms of k, a˜ and b˜.a˜ and b˜.
(c) Using →AQ=→AR+→RQ,−−→AQ=−−→AR+−−→RQ, find the value of h and of k.
Solution:
(a)(i)
→AP=→AO+→OP→AP=−→OA+→OP→AP=−8a˜+4b˜−−→AP=−−→AO+−−→OP−−→AP=−−−→OA+−−→OP−−→AP=−8a˜+4b˜
(a)(ii)
→OQ=→OA+→AQ→OQ=8a˜+14→AB→OQ=8a˜+14(→AO+→OB)→OQ=8a˜+14(−8a˜+4→OP)→OQ=8a˜+14(−8a˜+4(4b˜))→OQ=8a˜−2a˜+4b˜→OQ=6a˜+4b˜−−→OQ=−−→OA+−−→AQ−−→OQ=8a˜+14−−→AB−−→OQ=8a˜+14(−−→AO+−−→OB)−−→OQ=8a˜+14(−8a˜+4−−→OP)−−→OQ=8a˜+14(−8a˜+4(4b˜))−−→OQ=8a˜−2a˜+4b˜−−→OQ=6a˜+4b˜
(b)(i)
→AR=h→AP→AR=h(−8a˜+4b˜)→AR=−8ha˜+4hb˜−−→AR=h−−→AP−−→AR=h(−8a˜+4b˜)−−→AR=−8ha˜+4hb˜
(b)(ii)
→RQ=k→OQ→RQ=k(6a˜+4b˜)→RQ=6ka˜+4kb˜−−→RQ=k−−→OQ−−→RQ=k(6a˜+4b˜)−−→RQ=6ka˜+4kb˜
(c)
→AQ=→AR+→RQ→AQ=−8ha˜+4hb˜+(6ka˜+4kb˜)→AO+→OQ=−8ha˜+4hb˜+6ka˜+4kb˜−8a˜+6a˜+4b˜=−8ha˜+6ka˜+4hb˜+4kb˜−2a˜+4b˜=−8ha˜+6ka˜+4hb˜+4kb˜−2=−8h+6k−1=−4h+3k→(1)4=4h+4k1=h+kk=1−h→(2)Substitute (2) into (1),−1=−4h+3(1−h)−1=−4h+3−3h−4=−7hh=47From (2),k=1−47=37−−→AQ=−−→AR+−−→RQ−−→AQ=−8ha˜+4hb˜+(6ka˜+4kb˜)−−→AO+−−→OQ=−8ha˜+4hb˜+6ka˜+4kb˜−8a˜+6a˜+4b˜=−8ha˜+6ka˜+4hb˜+4kb˜−2a˜+4b˜=−8ha˜+6ka˜+4hb˜+4kb˜−2=−8h+6k−1=−4h+3k→(1)4=4h+4k1=h+kk=1−h→(2)Substitute (2) into (1),−1=−4h+3(1−h)−1=−4h+3−3h−4=−7hh=47From (2),k=1−47=37
Question 2:
Given that →AB=(1014),→OB=(46)−−→AB=(1014),−−→OB=(46) and →CD=(m7)−−→CD=(m7) , find
(a) the coordinates of A,
(b) the unit vector in the direction of →OA−−→OA .
(c) the value of m if CD is parallel to AB .
Solution:
(a)
→AB=→AO+→OB(1014)=(xy)+(46)(xy)=(1014)−(46)→AO=(68)→OA=(−6−8)A=(−6,−8)−−→AB=−−→AO+−−→OB(1014)=(xy)+(46)(xy)=(1014)−(46)−−→AO=(68)−−→OA=(−6−8)A=(−6,−8)
(b)
|→OA|=√(−6)2+(−8)2|→OA|=√100=10the unit vector in the direction of →OA=→OA|→OA|=(−6−8)10=110(−6−8)=(−35−45)∣∣∣−−→OA∣∣∣=√(−6)2+(−8)2∣∣∣−−→OA∣∣∣=√100=10the unit vector in the direction of −−→OA=−−→OA∣∣∣−−→OA∣∣∣=(−6−8)10=110(−6−8)=(−35−45)
(c)
Given →CD parallel →AB∴ →CD=k→AB(m7)=k(1014)(m7)=(10k14k)7=14kk=12m=10k=10(12)=5
Given that →AB=(1014),→OB=(46)−−→AB=(1014),−−→OB=(46) and →CD=(m7)−−→CD=(m7) , find
(a) the coordinates of A,
(b) the unit vector in the direction of →OA−−→OA .
(c) the value of m if CD is parallel to AB .
Solution:
(a)
→AB=→AO+→OB(1014)=(xy)+(46)(xy)=(1014)−(46)→AO=(68)→OA=(−6−8)A=(−6,−8)−−→AB=−−→AO+−−→OB(1014)=(xy)+(46)(xy)=(1014)−(46)−−→AO=(68)−−→OA=(−6−8)A=(−6,−8)
(b)
|→OA|=√(−6)2+(−8)2|→OA|=√100=10the unit vector in the direction of →OA=→OA|→OA|=(−6−8)10=110(−6−8)=(−35−45)∣∣∣−−→OA∣∣∣=√(−6)2+(−8)2∣∣∣−−→OA∣∣∣=√100=10the unit vector in the direction of −−→OA=−−→OA∣∣∣−−→OA∣∣∣=(−6−8)10=110(−6−8)=(−35−45)
(c)
Given →CD parallel →AB∴ →CD=k→AB(m7)=k(1014)(m7)=(10k14k)7=14kk=12m=10k=10(12)=5