Question 3:
(a) Sketch the graph of y=32cos2x for 0≤x≤32π.y=32cos2x for 0≤x≤32π.
(b) Hence, using the same axes, sketch a suitable straight line to find the number of solutions to the equation 43πx−cos2x=32 for 0≤x≤32π43πx−cos2x=32 for 0≤x≤32π
State the number of solutions.
Solution:
(a)(b)
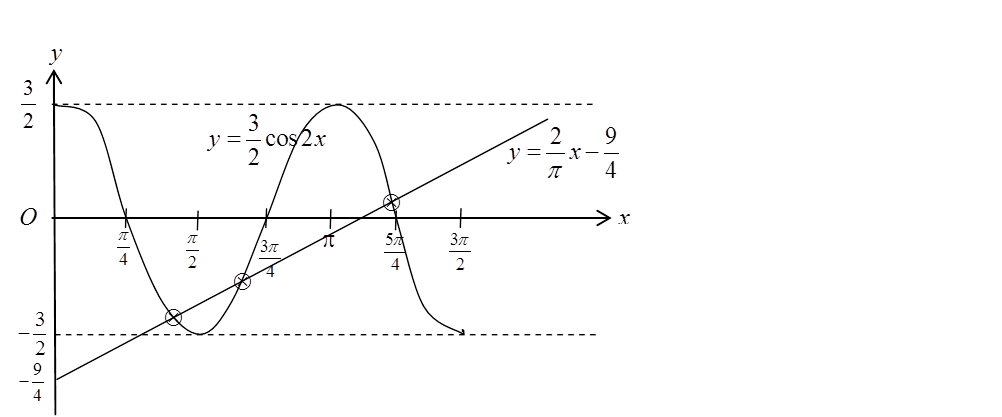
43πx−cos2x=32cos2x=43πx−3232cos2x=32(43πx−32)y=2πx−94To sketch the graph of y=2πx−94x=0, y=−94x=3π2, y=34Number of solutions =Number of intersection points= 3
(a) Sketch the graph of y=32cos2x for 0≤x≤32π.y=32cos2x for 0≤x≤32π.
(b) Hence, using the same axes, sketch a suitable straight line to find the number of solutions to the equation 43πx−cos2x=32 for 0≤x≤32π43πx−cos2x=32 for 0≤x≤32π
State the number of solutions.
Solution:
(a)(b)

Question 4:
(a)Prove that(cosecx−secxsecxcosecx)2=1−sin2x
[3 marks]
(b)(i) Sketch the graph of y = 1 – sin2x for 0 ≤ x ≤ 2π.
(b)(ii) Hence, using the same axes, sketch a suitable straight line to find the number of solutions to the equation 2−(cosecx−secxsecxcosecx)2=xπfor 0 ≤ x ≤ 2π.
State the number of soultions.
[7 marks]
[7 marks]
Solution:
(a)
LHS=(cosecx−secxsecxcosecx)2=(cosecxsecxcosecx−secxsecxcosecx)2=(1secx−1cosecx)2=(cosx−sinx)2=cos2x−2cosxsinx+sin2x=1−sin2x(RHS)
(b)(i)
(b)(ii)
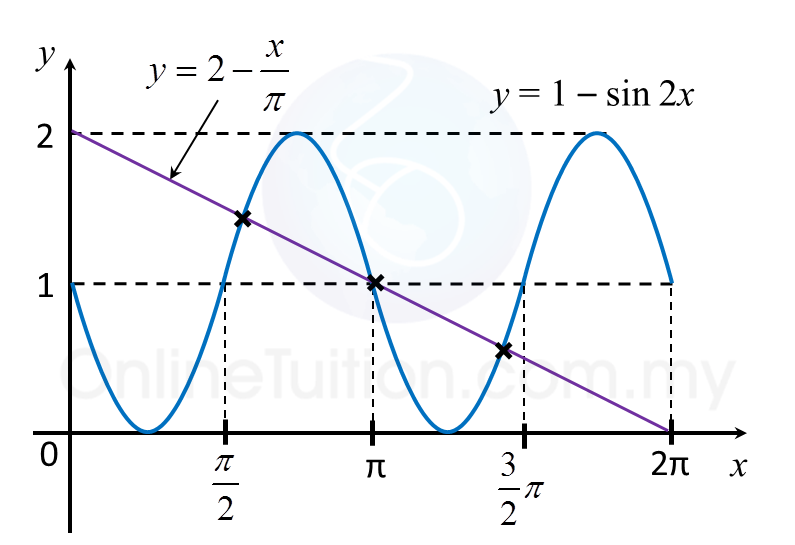
2−(cosecx−secxsecxcosecx)2=xπ2−(1−sin2x)=xπ←From 4(a)2−y=xπ←From 4(b)(i)y=2−xπ(suitable straight line)
2−(cosecx−secxsecxcosecx)2=xπ2−(1−sin2x)=xπ←From 4(a)2−y=xπ←From 4(b)(i)y=2−xπ(suitable straight line)
x |
0 |
π |
2π |
y |
2 |
1 |
0 |
From the graph, there is 3 number of solutions.