Question 6:
(a) Diagram 5 shows the graph of the function f(x)=|x−3|+1 for domain −2⩽x⩽7.
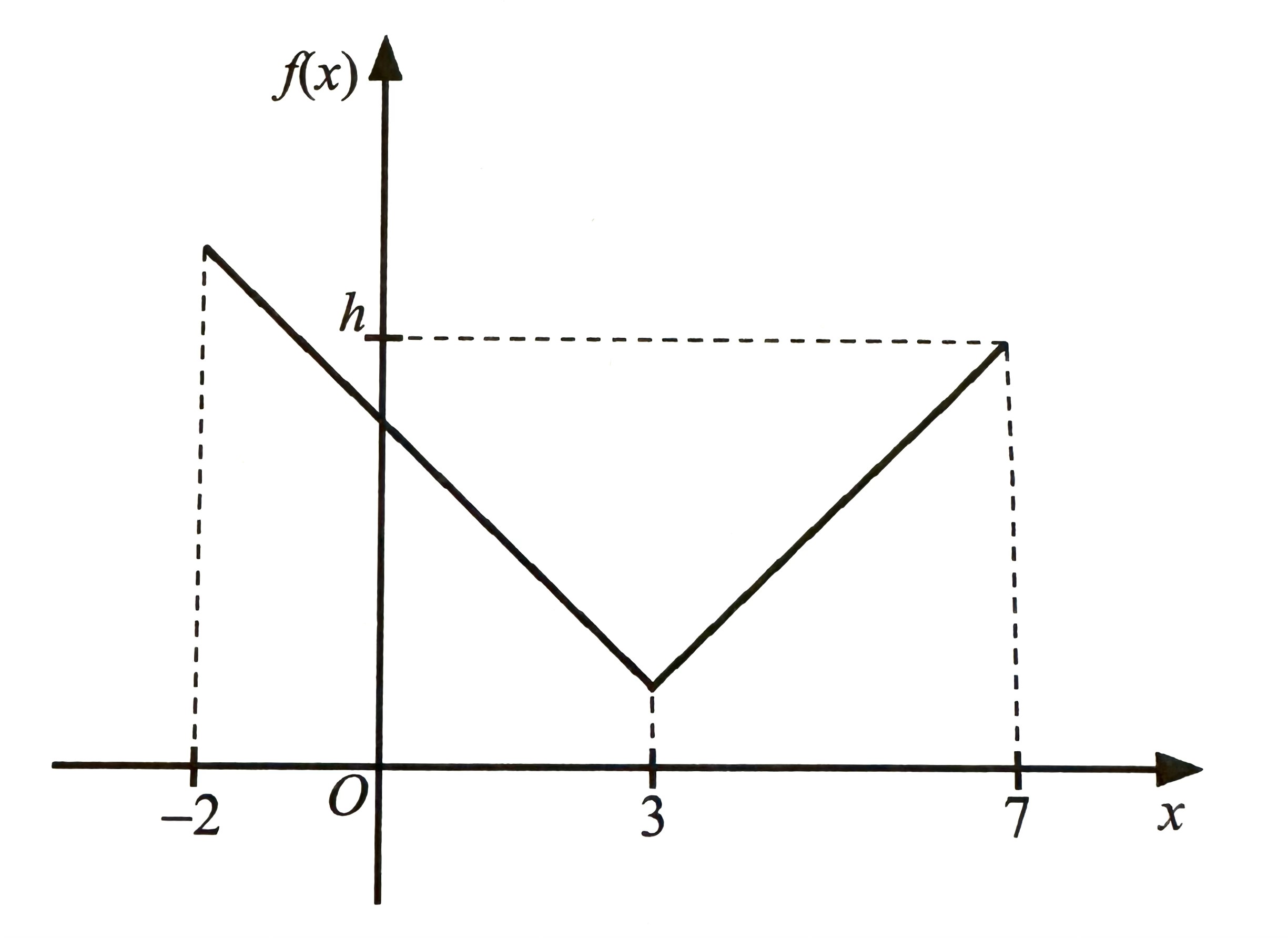
Find the value of the other object of h. [2 marks]
(b) Given that the functions f:x→4x−1 and g:x→2x+3, find
(i) the value of p if f −1(p)=2,
(ii) the value of x if fg(x)=5f(2). [4 marks]
Solution:
(a)
f(7)=h|7−3|+1=h|4|+1=hh=5
f(x)=5|x−3|+1=5|x−3|=4
x−3=4x=7
x−3=−4x=−1
∴ Object of h is −1.
(b)(i)
f(x)=4x−1 Let 4x−1=y4x=y+1x=y+14f1(x)=x+14
f1(p)=2p+14=2p+1=8p=7
(b)(ii)
fg(x)=5f(2)f(2x+3)=5[4(2)−1]4(2x+3)−1=5(8−1)8x+12−1=5(7)8x+11=358x=24x=3
(a) Diagram 5 shows the graph of the function f(x)=|x−3|+1 for domain −2⩽x⩽7.

Find the value of the other object of h. [2 marks]
(b) Given that the functions f:x→4x−1 and g:x→2x+3, find
(i) the value of p if f −1(p)=2,
(ii) the value of x if fg(x)=5f(2). [4 marks]
Solution:
(a)
f(7)=h|7−3|+1=h|4|+1=hh=5
f(x)=5|x−3|+1=5|x−3|=4
x−3=4x=7
x−3=−4x=−1
∴ Object of h is −1.
(b)(i)
f(x)=4x−1 Let 4x−1=y4x=y+1x=y+14f1(x)=x+14
f1(p)=2p+14=2p+1=8p=7
(b)(ii)
fg(x)=5f(2)f(2x+3)=5[4(2)−1]4(2x+3)−1=5(8−1)8x+12−1=5(7)8x+11=358x=24x=3
Question 7:
(a) Diagram 6 shows a curve and a tangent to the curve.
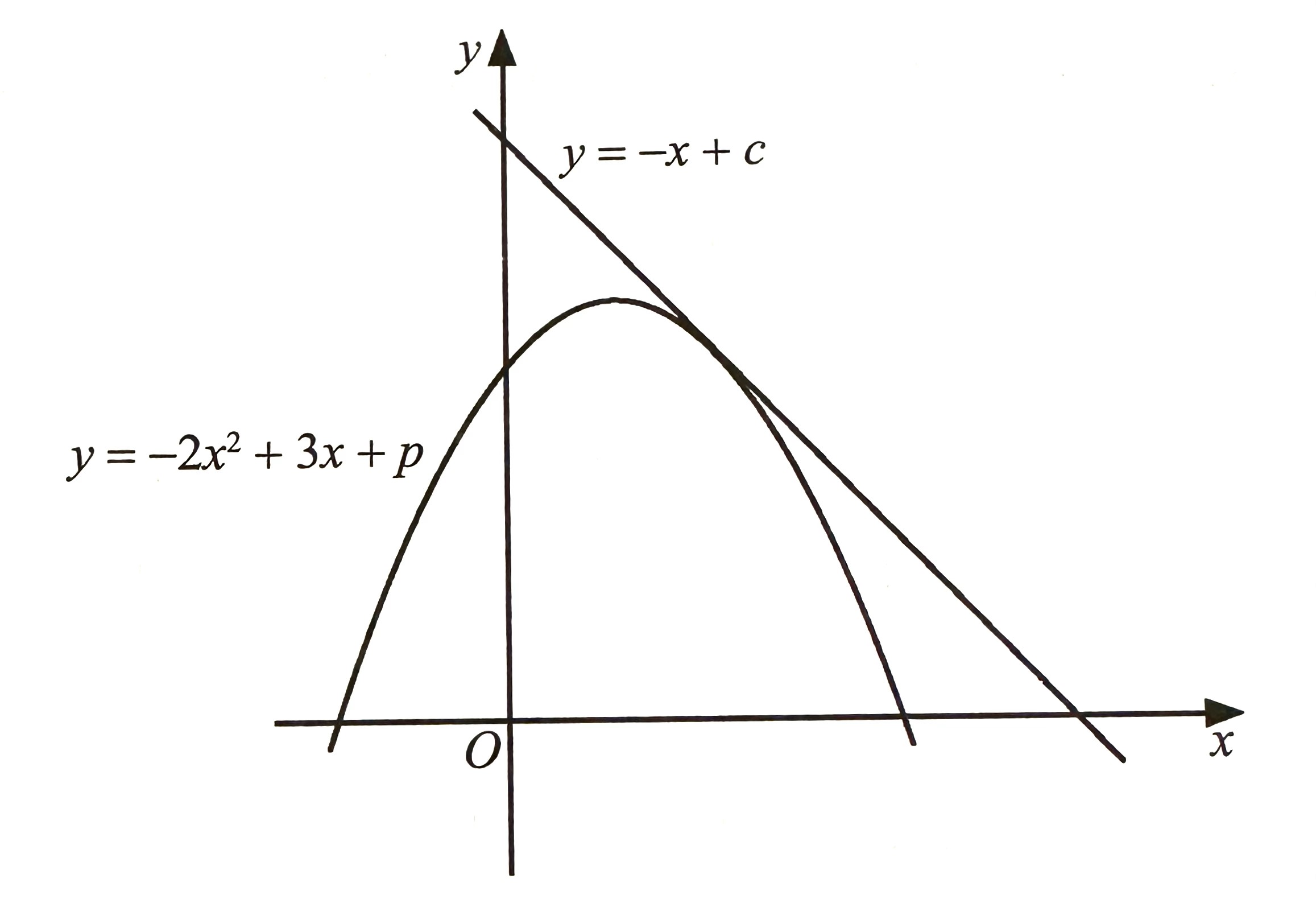 Express p in terms of c.
Express p in terms of c.
[3 marks]
(b) Find the range of values of x for (x + 1)(-3x – 3) < (x – 1) by using the table method.
[2 marks]
(c) It is given that p and 2p are the roots of the quadratic equation 2x2 + 6x + 4p2 = 0.
Find the quadratic equation with roots (p – 1) and (p + 1).
[3 marks]
Solution:
(a)
y=−x+c…(1)y=−2x2+3x+p…(2)(1)=(2):−2x2+3x+p=−x+c−2x2+3x+p+x−c=02x2−4x−p+c=0a=2,b=−4,c=−p+c
Tangent to the curve, b2−4ac=0(−4)2−4(2)(−p+c)=016−8(−p+c)=016+8p−8c=08p=8c−16p=c−2
(b)
(x + 1)(-3x – 3) < x – 1
-3x2 – 3x – 3x – 3 – x + 1 < 0
-3x2 – 7x -2 < 0
3x2 – 7x – 2 > 0
(3x + 1)(x + 2) > 0
When (3x + 1)(x + 2) = 0,
x = -1/3 or -2
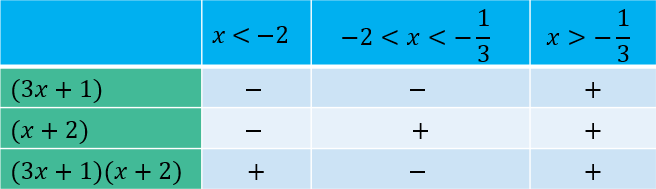
From the table,
(3x + 1)(x + 2) > 0 when x < -2 or x > -1/3
∴ x < -2 or x > -1/3
(c)
2x2+6x+4p2=0 Roots =p,2p SOR: p+2p=−623p=−3p=−1
New roots: p−1=−1−1,=−2p+1=−1+1=0
SOR: −2+0=−2 POR: (−2)(0)=0
x2−(SOR)x+(POR)=0x2−(−2)x+0=0x2+4x=0
(a) Diagram 6 shows a curve and a tangent to the curve.
 Express p in terms of c.
Express p in terms of c. [3 marks]
(b) Find the range of values of x for (x + 1)(-3x – 3) < (x – 1) by using the table method.
[2 marks]
(c) It is given that p and 2p are the roots of the quadratic equation 2x2 + 6x + 4p2 = 0.
Find the quadratic equation with roots (p – 1) and (p + 1).
[3 marks]
Solution:
(a)
y=−x+c…(1)y=−2x2+3x+p…(2)(1)=(2):−2x2+3x+p=−x+c−2x2+3x+p+x−c=02x2−4x−p+c=0a=2,b=−4,c=−p+c
Tangent to the curve, b2−4ac=0(−4)2−4(2)(−p+c)=016−8(−p+c)=016+8p−8c=08p=8c−16p=c−2
(b)
(x + 1)(-3x – 3) < x – 1
-3x2 – 3x – 3x – 3 – x + 1 < 0
-3x2 – 7x -2 < 0
3x2 – 7x – 2 > 0
(3x + 1)(x + 2) > 0
When (3x + 1)(x + 2) = 0,
x = -1/3 or -2

From the table,
(3x + 1)(x + 2) > 0 when x < -2 or x > -1/3
∴ x < -2 or x > -1/3
(c)
2x2+6x+4p2=0 Roots =p,2p SOR: p+2p=−623p=−3p=−1
New roots: p−1=−1−1,=−2p+1=−1+1=0
SOR: −2+0=−2 POR: (−2)(0)=0
x2−(SOR)x+(POR)=0x2−(−2)x+0=0x2+4x=0