Question 4:
Diagram 3 shows point A lies on the circumference of a unit circle.
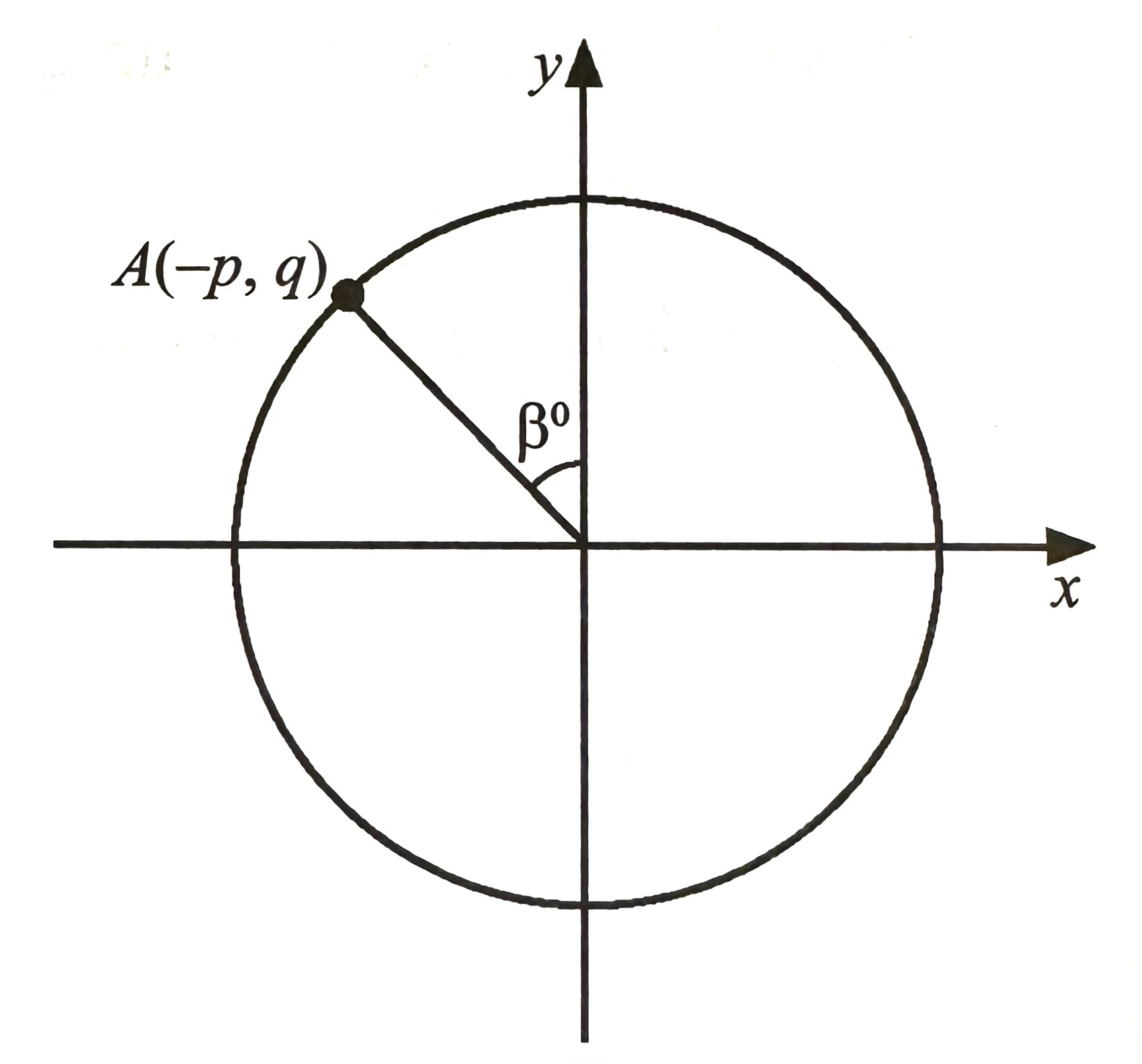 Express in terms of p and / or q of.
Express in terms of p and / or q of.
(a) tan β,
(b) cot (180o + β)
[2 marks]
Solution:
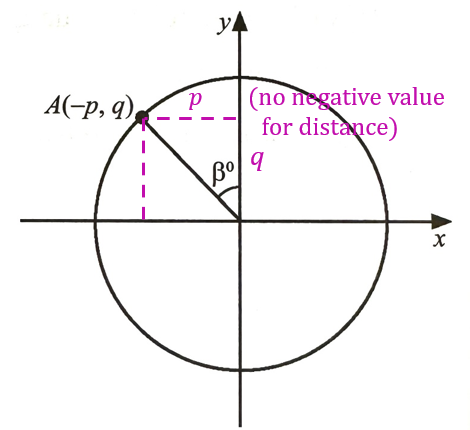
(a)
tanβ= Opposite Adjacent (TOA)tanβ=pq
(b)
tan(A+B)=tanA+tanB1−tanAtanBcot(180∘+β)=1tan(180∘+β)=1tan180∘+tanβ1−tan180∘tanβ=10+tanβ1−(0)tanβ=1tanβ1=1tanβ=1pq=qp
Diagram 3 shows point A lies on the circumference of a unit circle.
 Express in terms of p and / or q of.
Express in terms of p and / or q of.(a) tan β,
(b) cot (180o + β)
[2 marks]
Solution:

(a)
tanβ= Opposite Adjacent (TOA)tanβ=pq
(b)
tan(A+B)=tanA+tanB1−tanAtanBcot(180∘+β)=1tan(180∘+β)=1tan180∘+tanβ1−tan180∘tanβ=10+tanβ1−(0)tanβ=1tanβ1=1tanβ=1pq=qp
Question 5:
Diagram 4 shows three points on a Cartesian plane.
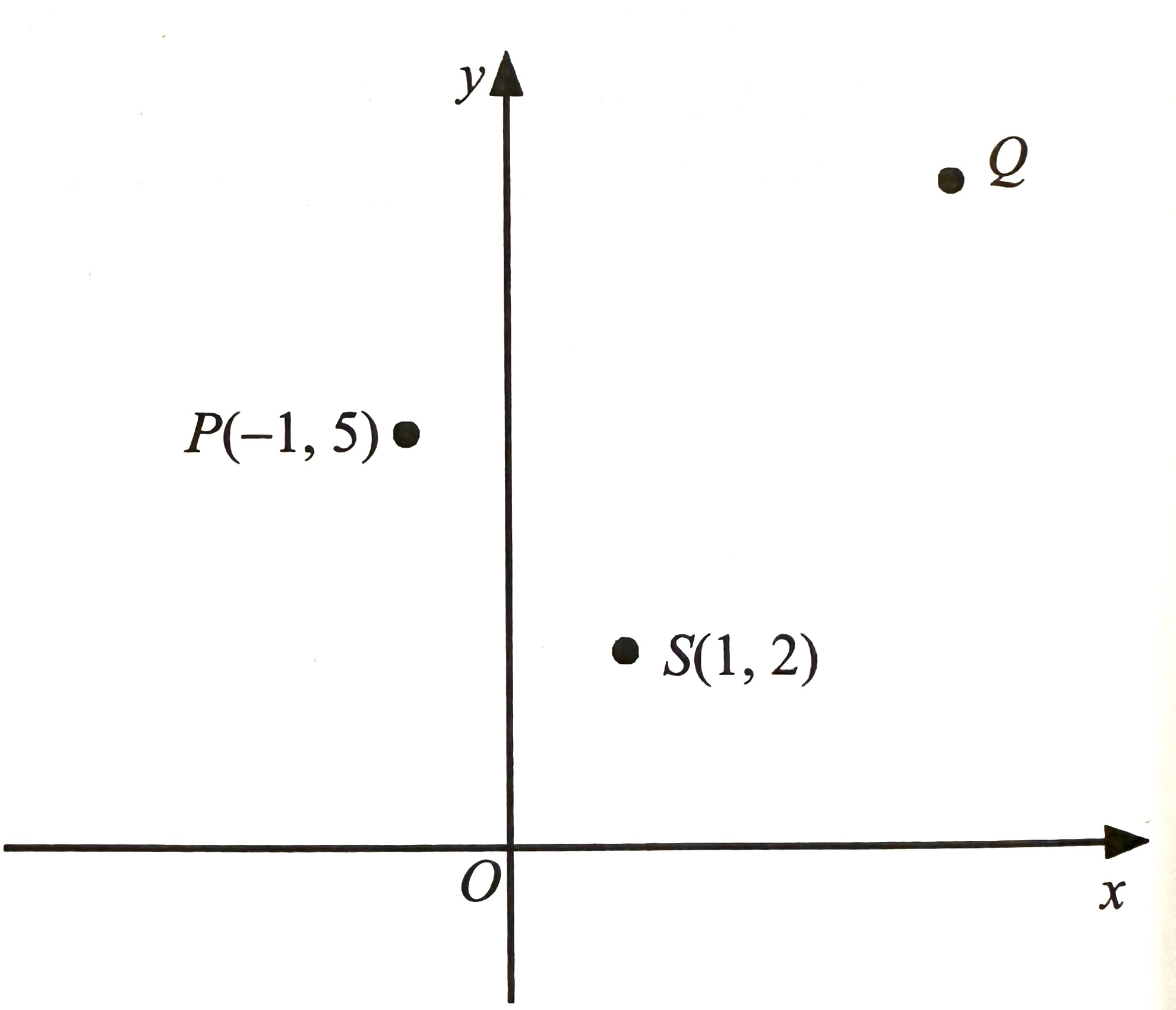 (a) State →SP [1 mark].
(a) State →SP [1 mark].
(b) It is given that →PQ=5i_+mj_.
(i) If →SP+3→PQ=13i_+15j, find the value of m by using vector’s arithmetic operations
(ii) Hence, determine the unit vector in the direction of →PQ.
[4 marks]
Solution:
(a)
→SP=→SO+→OP=−(i+2j∼)+(−i+5j∼)=−i−2j∼−i∼+5j∼=−2i∼+3j∼
(b)(i)
→SP+3→PQ=13i_+15j_(−2i_+3j_)+3(5i_+mj_)=13i_+15j_−2i_+3j_+15i_+3mj_)=13i_+15j_13i_+(3m+3)j_=13i_+15j_
3m+3=153m=12m=4
(b)(ii)
→PQ=5i_+4j_|→PQ|=√52+42|→PQ|=√41
Unit vector =5i_+4j_√41
Diagram 4 shows three points on a Cartesian plane.
 (a) State →SP [1 mark].
(a) State →SP [1 mark]. (b) It is given that →PQ=5i_+mj_.
(i) If →SP+3→PQ=13i_+15j, find the value of m by using vector’s arithmetic operations
(ii) Hence, determine the unit vector in the direction of →PQ.
[4 marks]
Solution:
(a)
→SP=→SO+→OP=−(i+2j∼)+(−i+5j∼)=−i−2j∼−i∼+5j∼=−2i∼+3j∼
(b)(i)
→SP+3→PQ=13i_+15j_(−2i_+3j_)+3(5i_+mj_)=13i_+15j_−2i_+3j_+15i_+3mj_)=13i_+15j_13i_+(3m+3)j_=13i_+15j_
3m+3=153m=12m=4
(b)(ii)
→PQ=5i_+4j_|→PQ|=√52+42|→PQ|=√41
Unit vector =5i_+4j_√41