Question 4:
Diagram 2 shows the curve y = 4x – x2 and tangent to the curve at point Q passes point P.
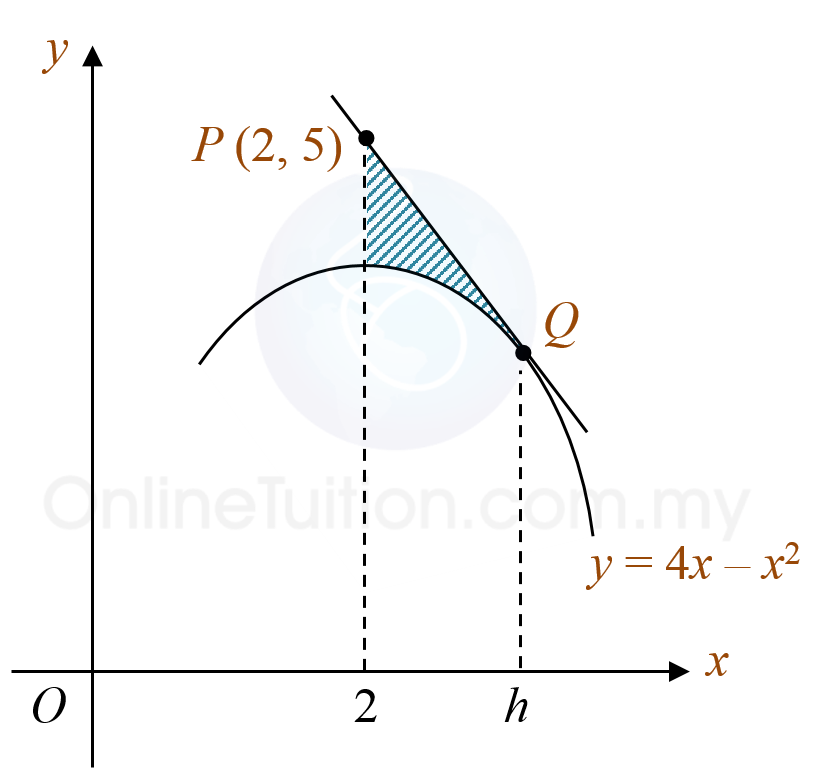Diagram 2
(a) Show that h = 3. [4 marks]
(b) Calculate the area of the shaded region. [4 marks]
Solution:
(a)
y=4x−x2dydx=4−2xAt point Q(h, 4h−h2)dydx=4−2hEquation of tangent at Qy−y1=dydx(x−x1)y−(4h−h2)=(4−2h)(x−h)
At point P(2, 5), x=2, y=55−4h+h2=(4−2h)(2−h)5−4h+h2=8−4h−4h+2h2h2−4h+3=0(h−1)(h−3)=0h=1 (rejected),h=3
(b)
Area of shaded region= Area of trapezium−Area under the curve=12(a+b)h−∫32y dx=12(5+3)1−∫324x−x2 dx=4−[4x22−x33]32=4−[18−9−(8−83)]=4−9+(8−83)=13 unit2
Diagram 2 shows the curve y = 4x – x2 and tangent to the curve at point Q passes point P.
Diagram 2(a) Show that h = 3. [4 marks]
(b) Calculate the area of the shaded region. [4 marks]
Solution:
(a)
y=4x−x2dydx=4−2xAt point Q(h, 4h−h2)dydx=4−2hEquation of tangent at Qy−y1=dydx(x−x1)y−(4h−h2)=(4−2h)(x−h)
At point P(2, 5), x=2, y=55−4h+h2=(4−2h)(2−h)5−4h+h2=8−4h−4h+2h2h2−4h+3=0(h−1)(h−3)=0h=1 (rejected),h=3
(b)
Area of shaded region= Area of trapezium−Area under the curve=12(a+b)h−∫32y dx=12(5+3)1−∫324x−x2 dx=4−[4x22−x33]32=4−[18−9−(8−83)]=4−9+(8−83)=13 unit2