Question 17 (3 marks):
It is given that ∫5(2x+3)ndx=p(2x+3)5+c , where c, n and p are constants.
Find the value of n and of p.
Solution:
∫5(2x+3)ndx=∫5(2x+3)−ndx=5(2x+3)−n+1(−n+1)×2+c=52(1−n)×1(2x+3)n−1+c=52(1−n)(2x+3)n−1+cCompare 52(1−n)(2x+3)n−1with p(2x+3)5n−1=5n=652(1−n)=p52(1−6)=p52(−5)=pp=−12
It is given that ∫5(2x+3)ndx=p(2x+3)5+c , where c, n and p are constants.
Find the value of n and of p.
Solution:
∫5(2x+3)ndx=∫5(2x+3)−ndx=5(2x+3)−n+1(−n+1)×2+c=52(1−n)×1(2x+3)n−1+c=52(1−n)(2x+3)n−1+cCompare 52(1−n)(2x+3)n−1with p(2x+3)5n−1=5n=652(1−n)=p52(1−6)=p52(−5)=pp=−12
Question 18 (3 marks):
A straight line passes through P(3, 1) and Q(12, 7). The point R divides the line segment PQ such that 2PQ = 3RQ.
Find the coordinates of R.
Solution:
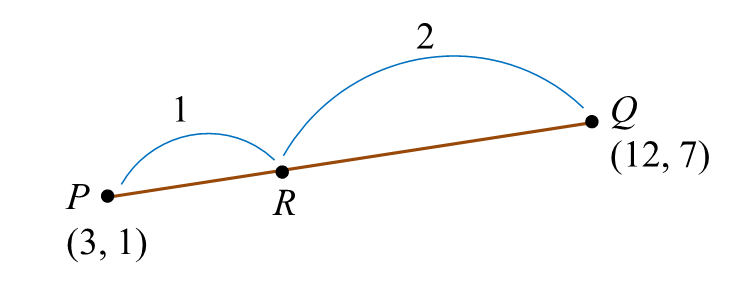
2PQ=3RQPQRQ=32Point R=(1(12)+2(3)1+2,1(7)+2(1)1+2)=(183,93)=(6,3)
A straight line passes through P(3, 1) and Q(12, 7). The point R divides the line segment PQ such that 2PQ = 3RQ.
Find the coordinates of R.
Solution:

2PQ=3RQPQRQ=32Point R=(1(12)+2(3)1+2,1(7)+2(1)1+2)=(183,93)=(6,3)
Question 19 (3 marks):
The variables x and y are related by the equation y=x+rx2 , where r is a constant. Diagram 8 shows a straight line graph obtained by plotting (y−x) against 1x2.
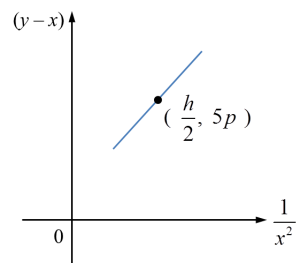 Diagram 8
Diagram 8
Express h in terms of p and r.
Solution:
y=x+rx2y−x=r(1x2)+0Y=mX+cm=r, c=0m=y2−y1x2−x1r=5p−0h2−0hr2=5phr=10ph=10pr
The variables x and y are related by the equation y=x+rx2 , where r is a constant. Diagram 8 shows a straight line graph obtained by plotting (y−x) against 1x2.
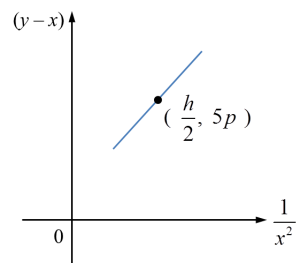 Diagram 8
Diagram 8Express h in terms of p and r.
Solution:
y=x+rx2y−x=r(1x2)+0Y=mX+cm=r, c=0m=y2−y1x2−x1r=5p−0h2−0hr2=5phr=10ph=10pr