Question 7:
Diagram below shows a curve y=14x2+3 which intersects the straight line y = x + 6 at point A.
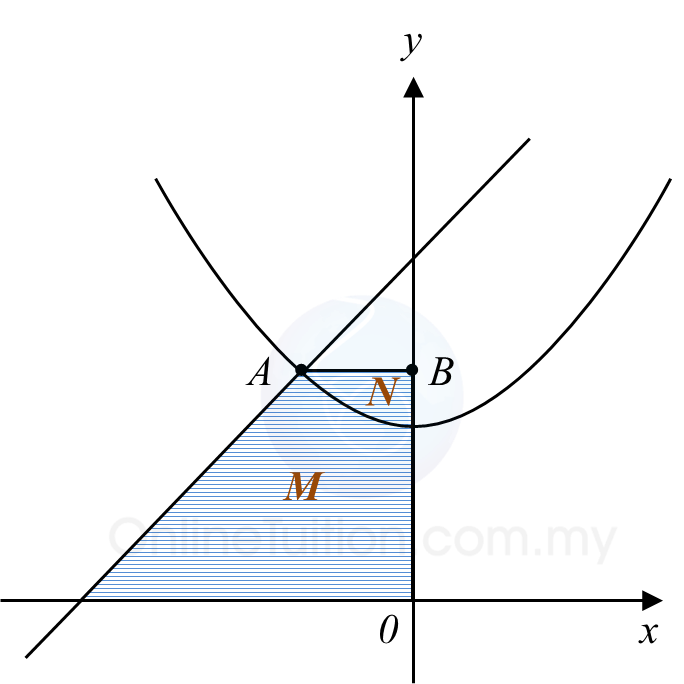
(a) Find the coordinates of A.
(b) Calculate
(i) the area of the shaded region M,
(ii) the volume generated, in terms of π, when the shaded region N is revolved 360o about the y-axis.
Solution:
(a)
y=14x2+3……….(1)y=x+6……….(2)Substitute (2) into (1),x+6=14x2+34x+24=x2+12x2−4x−12=0(x+2)(x−6)=0x=−2 or x=6 (rejected)When x=−2y=−2+6=4Therefore, A=(−2,4).
(b)(i)
At x-axis, y=0From y=x+6,x=−6Area of region M=Area of triangle+Area under the curve=12×(6−2)×4+∫0−2y dx=8+∫0−2(14x2+3) dx=8+[x34(3)+3x]0−2=8+[0−((−2)312+3(−2))]=8+[0−(−812−6)]=8+[0−(−203)]=1423 unit2
(b)(ii)
At y-axis, x=0, y=14(0)+3y=3y=14x2+34y=x2+12x2=4y−12Volume of N= π∫43x2dy= π∫43(4y−12)dy= π∫43(2y2−12y)dy=π[(2y2−12y)]43=π[(2(4)2−12(4))−(2(3)2−12(3))]=π(−16+18)=2π unit3
Diagram below shows a curve y=14x2+3 which intersects the straight line y = x + 6 at point A.

(a) Find the coordinates of A.
(b) Calculate
(i) the area of the shaded region M,
(ii) the volume generated, in terms of π, when the shaded region N is revolved 360o about the y-axis.
Solution:
(a)
y=14x2+3……….(1)y=x+6……….(2)Substitute (2) into (1),x+6=14x2+34x+24=x2+12x2−4x−12=0(x+2)(x−6)=0x=−2 or x=6 (rejected)When x=−2y=−2+6=4Therefore, A=(−2,4).
(b)(i)
At x-axis, y=0From y=x+6,x=−6Area of region M=Area of triangle+Area under the curve=12×(6−2)×4+∫0−2y dx=8+∫0−2(14x2+3) dx=8+[x34(3)+3x]0−2=8+[0−((−2)312+3(−2))]=8+[0−(−812−6)]=8+[0−(−203)]=1423 unit2
(b)(ii)
At y-axis, x=0, y=14(0)+3y=3y=14x2+34y=x2+12x2=4y−12Volume of N= π∫43x2dy= π∫43(4y−12)dy= π∫43(2y2−12y)dy=π[(2y2−12y)]43=π[(2(4)2−12(4))−(2(3)2−12(3))]=π(−16+18)=2π unit3
Question 8:
Diagram below shows the curve y=4x2 and the straight line y = mx + c. The straight line y = mx + c is a tangent to the curve at (2, 1).

(a) Find the value of m and of c.
(b) Calculate the area of the shaded region.
(c) It is given that the volume generated when the region bounded by the curve, the x–axis and the straight lines x = 2 and x = h is revolved through 360o about the x-axis is 38π81 unit3.
Find the value of h, such that h > 2.
Solution:
(a)
y=4x2=4x−2dydx=−8x−3=−8x3At x=2,dydx=−823=−1Equation of tangent:y−y1=m(x−x1)y−1=−1(x−2)y=−x+2+1y=−x+3m=−1, c=3
(b)
At x-axis, y=0From the straight line y=−x+3,x=3Area of the shaded region=Area under the curve−Area of triangle=∫42y dx−12×1×1=∫42(4x−2) dx−12=[4x−1−1]42−12=[−4x]42−12=[−44−(−42)]−12=12 unit2
(c)
Volume generated=38π81π∫h2y2 dx=38π81∫h2(4x2)2dx=3881∫h2(16x4)dx=3881∫h2(16x−4)dx=3881[16x−3−3]h2=3881[−163x3]h2=3881−163h3−(−163(2)3)=3881163h3=1624−3881163h3=16813h3=81h3=27h=3
Diagram below shows the curve y=4x2 and the straight line y = mx + c. The straight line y = mx + c is a tangent to the curve at (2, 1).

(a) Find the value of m and of c.
(b) Calculate the area of the shaded region.
(c) It is given that the volume generated when the region bounded by the curve, the x–axis and the straight lines x = 2 and x = h is revolved through 360o about the x-axis is 38π81 unit3.
Find the value of h, such that h > 2.
Solution:
(a)
y=4x2=4x−2dydx=−8x−3=−8x3At x=2,dydx=−823=−1Equation of tangent:y−y1=m(x−x1)y−1=−1(x−2)y=−x+2+1y=−x+3m=−1, c=3
(b)
At x-axis, y=0From the straight line y=−x+3,x=3Area of the shaded region=Area under the curve−Area of triangle=∫42y dx−12×1×1=∫42(4x−2) dx−12=[4x−1−1]42−12=[−4x]42−12=[−44−(−42)]−12=12 unit2
(c)
Volume generated=38π81π∫h2y2 dx=38π81∫h2(4x2)2dx=3881∫h2(16x4)dx=3881∫h2(16x−4)dx=3881[16x−3−3]h2=3881[−163x3]h2=3881−163h3−(−163(2)3)=3881163h3=1624−3881163h3=16813h3=81h3=27h=3