9.3 Area of Triangle

9.3 Area of Triangle

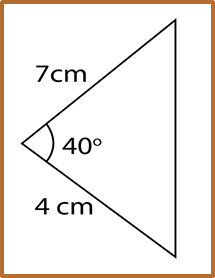
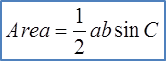Example:
Calculate the area of the triangle above.

Solution:
$\begin{array}{l}Area=\frac{1}{2}ab\sin C\\ Area=\frac{1}{2}(7)(4)\sin {40}^o\\ Area=9{\text{ cm}}^2\end{array}$

Example:

Diagram above shows a triangle ABC, where AC = 8 cm and ∠C = 32^o. Point D lies on straight line BC where BD = 10 cm and ∠ADB = 70^o . Calculate
(a) the length, in cm, of CD,
(b) the area, in cm² of ∆ ADC,
(c) the area, in cm² of ∆ ABC,
(d) the length, in cm, of AB.

Solution:
(a)
$\begin{array}{l}\angle ADC+{70}^o={180}^o\\ \angle ADC={110}^o\\ \angle CAD={180}^o-{110}^o-{32}^o\\ \angle CAD={38}^o\\ \\ \text{Using Sine Rule,}\\ \frac{8}{\sin {110}^o}=\frac{CD}{\sin {38}^o}\\ CD\sin {110}^o=8\sin {38}^o\\ CD\left(0.940\right)=8\left(0.616\right)\\ CD=5.243\end{array}$

(b)
$\begin{array}{l}\text{Area of }△ADC\\ =\frac{1}{2}\left(8\right)\left(5.243\right)\text{sin}{32}^o\\ =20.972\left(0.530\right)\\ =11.12{\text{ cm}}^2\end{array}$

(c)
$\begin{array}{l}\text{Area of }△ABC\\ =\frac{1}{2}\left(8\right)\left(10+5.243\right)\text{sin}{32}^o\\ =60.972\left(0.530\right)\\ =32.315{\text{ cm}}^2\end{array}$

(d)
$\begin{array}{l}\text{Use Cosine Rule for }△ABC,\\ A{B}^2={15.243}^2+8^2-2\left(15.243\right)\left(8\right)\cos {32}^o\\ A{B}^2=232.35+64-206.83\\ A{B}^2=89.52\\ AB=9.462\text{ cm}\end{array}$