Question 9:
Given that the quadratic function f(x) = 2x2 – px + p has a minimum value of –18 at x = 1.
(a) Find the values of p and q.
(b) With the value of p and q found in (a), find the values of x, where graph f(x) cuts the x-axis.
(c) Hence, sketch the graph of f(x).
Solution:
(a)
f(x)=2x2−px+q=2[x2−p2x+q2]=2[(x+−p4)2−(−p4)2+q2]=2[(x−p4)2−p216+q2]=2(x−p4)2−p28+q
∴p4=1−−−−−−(1)and −p28+q=−18−−−(2)From(1),p=4.Substitute p=4 into (2):−(4)28+q=−18 −168+q=−18 q=−18+2 =−16
(b)
f(x)=2x2−4x−16f(x)=0 when it cuts x-axis2x2−4x−16=0x2−2x−8=0(x−4)(x+2)=0x=4,−2Graph f(x) cuts x-axis at x=−2 and x=4.
(c)

Given that the quadratic function f(x) = 2x2 – px + p has a minimum value of –18 at x = 1.
(a) Find the values of p and q.
(b) With the value of p and q found in (a), find the values of x, where graph f(x) cuts the x-axis.
(c) Hence, sketch the graph of f(x).
Solution:
(a)
f(x)=2x2−px+q=2[x2−p2x+q2]=2[(x+−p4)2−(−p4)2+q2]=2[(x−p4)2−p216+q2]=2(x−p4)2−p28+q
∴p4=1−−−−−−(1)and −p28+q=−18−−−(2)From(1),p=4.Substitute p=4 into (2):−(4)28+q=−18 −168+q=−18 q=−18+2 =−16
(b)
f(x)=2x2−4x−16f(x)=0 when it cuts x-axis2x2−4x−16=0x2−2x−8=0(x−4)(x+2)=0x=4,−2Graph f(x) cuts x-axis at x=−2 and x=4.
(c)

Question 10:
(a) Find the range of values of k if the equation x2 – kx + 3k – 5 = 0 does not have real roots.
(b) Show that the quadratic equation hx2 – (h + 3)x + 1 = 0 has real and distinc roots for all values of h.
Solution:
(a)
x2−kx+(3k−5)=0If the above equation has no real root,∴ b2−4ac<0.k2−4(3k−5)<0k2−12k+20<0(k−2)(k−10)<0
Graph function y = (k – 2)(k – 10) cuts the horizontal line at k = 2 and k = 10 when b2 – 4ac < 0.
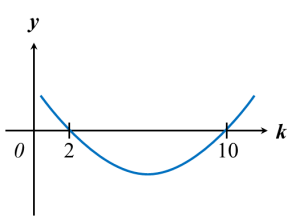
The range of values of k that satisfy the inequality above is 2 < k < 10.
(b)
hx2−(h+3)x+1=0b2−4ac=(h+3)2−4(h)(1)=h2+6h+9−4h=h2+2h+9=(h+22)2−(22)2+9=(h+1)2−1+9=(h+1)2+8
The minimum value of (h + 1) + 8 is 8, a positive value. Therefore, b2 – 4ac > 0 for all values of h.
Hence, quadratic equation hx2 – (h + 3)x + 1 = 0 has real and distinc roots for all values of h.
(a) Find the range of values of k if the equation x2 – kx + 3k – 5 = 0 does not have real roots.
(b) Show that the quadratic equation hx2 – (h + 3)x + 1 = 0 has real and distinc roots for all values of h.
Solution:
(a)
x2−kx+(3k−5)=0If the above equation has no real root,∴ b2−4ac<0.k2−4(3k−5)<0k2−12k+20<0(k−2)(k−10)<0
Graph function y = (k – 2)(k – 10) cuts the horizontal line at k = 2 and k = 10 when b2 – 4ac < 0.

The range of values of k that satisfy the inequality above is 2 < k < 10.
(b)
hx2−(h+3)x+1=0b2−4ac=(h+3)2−4(h)(1)=h2+6h+9−4h=h2+2h+9=(h+22)2−(22)2+9=(h+1)2−1+9=(h+1)2+8
The minimum value of (h + 1) + 8 is 8, a positive value. Therefore, b2 – 4ac > 0 for all values of h.
Hence, quadratic equation hx2 – (h + 3)x + 1 = 0 has real and distinc roots for all values of h.