8.3.2 Subtraction of Vectors
The subtraction of the vector
b˜
from the vector
a˜
is written as
a˜−b˜
. This operation can be considered as the addition of the vector
a˜
with the negative vector of
b˜
. Therefore
a˜−b˜=a˜+(−b˜).
Example 1:
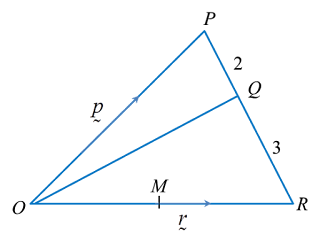
In the diagram above, vector →OP=p˜, →OR=r˜ and Q divides PR in the ratio of 2 : 3. Find the following vectors in terms of p˜ and r˜
(a) →PR(b) →OQ(c) →QM if M is the midpoint of OR.
Solution:
(a)
→PR=→PO+→OR=−→OP+→OR=−p˜+r˜
→PR=→PO+→OR=−→OP+→OR=−p˜+r˜
(b)
→OQ=→OP+→PQ=→OP+25→PR=p˜+25(−p˜+r˜)=p˜−25p˜+25r˜=35p˜+25r˜
(c)
→QM=→QO+→OM=−→OQ+→OM=−→OQ+12→OR=−(35p˜+25r˜)+12r˜=−35p˜−25r˜+12r˜=−35p˜+110r˜