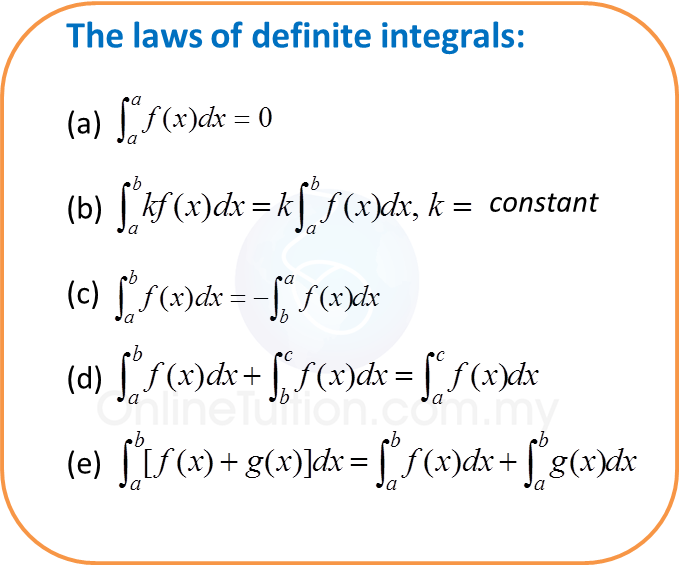
3.4b Laws of Definite Integrals
Example:
Given that
∫73f(x)dx=5
, find the values for each of the following:
(a)∫736f(x)dx(b)∫73[3−f(x)]dx(c)∫372f(x)dx(d)∫43f(x)dx+∫54f(x)dx+∫73f(x)dx(e)∫73f(x)+72dx
Solution:
(a)∫736f(x)dx=6∫73f(x)dx=6(5)=30(b)∫73[3−f(x)]dx=∫733dx−∫73f(x)dx=[3x]73−5=[3(7)−3(3)]−5=7(c)∫372f(x)dx=−∫732f(x)dx=−2∫73f(x)dx=−2(5)=−10(d)∫43f(x)dx+∫54f(x)dx+∫73f(x)dx=∫73f(x)dx=5(e)∫73f(x)+72dx=∫73[12f(x)+72]dx=∫7312f(x)dx+∫7372dx=12∫73f(x)dx+[7x2]73=12(5)+[7(7)2−7(3)2]=52+14=1612



These information are much helpful!Thank You.