3.4a Definite Integral of f(x) from x=a to x=b

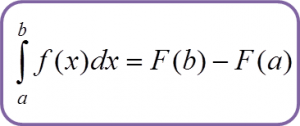
3.4a Definite Integral of f(x) from x=a to x=b

Example:

Evaluate each of the following.

\begin{array}{l} (a) \int_{- 1}^{0} (3 x^{2} - 2 x + 5) d x \\ (b) \int_{0}^{2} {(2 x + 1)}^{3} d x \end{array}

Solution:

\begin{array}{l} (a) \int_{- 1}^{0} (3 x^{2} - 2 x + 5) d x \\ = {[\frac{3 x^{3}}{3} - \frac{2 x^{2}}{2} + 5 x]}_{- 1}^{0} \\ = {[x^{3} - x^{2} + 5 x]}_{- 1}^{0} \\ = 0 - [{(- 1)}^{3} - {(- 1)}^{2} + 5 (- 1)] \\ = 0 - (- 1 - 1 - 5) \\ = 7 \\ (b) \int_{0}^{2} {(2 x + 1)}^{3} d x \\ = {[\frac{{(2 x + 1)}^{4}}{4 (2)}]}_{0}^{2} \\ = {[\frac{{(2 x + 1)}^{4}}{8}]}_{0}^{2} \\ = [\frac{{(2 (2) + 1)}^{4}}{8}] - [\frac{{(2 (0) + 1)}^{4}}{8}] \\ = \frac{625}{8} - \frac{1}{8} \\ = 78 \end{array}