Question 6:
Diagram 5 shows two curves x=√y and x=y28 are intersecting at point A. The straight line AB is parallel to the x-axis.
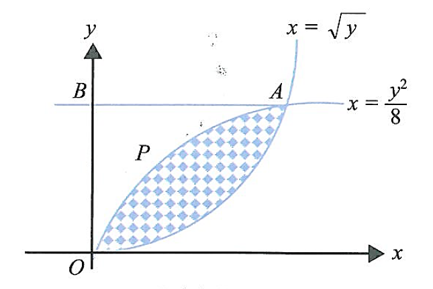
Find
(a) the equation of the straight line AB, [2 marks]
(b) area, in unit2, of the shaded region, [3 marks]
(c) the volume generated, in term of π, when the region P, which bounded by the curve x=y28 , the straight line AB and the y-axis, is revolved 360∘ about the y-axis. [3 marks]
Solution:
(a)
x=√y ….. (1)x=y28 ….. (2)Substitute (1) into (2),√y=y28(y12)2=(y28)2y=y46464=y4yy3=64y=4From (1),x=√4=2Point A=(2, 4)Equation of the straight line AB is y=4.
(b)
x=√y⇒y=x2x=y28⇒y=√8xArea of the shaded region=∫20(y1−y2)dx=∫20(√8x12−x2)dx=[√8x3232−x33]20=[2×2(2)12x323−x33]20=4(2)12(2)323−(2)33−0=163−83=83 unit2
(c)
x=y28⇒x2=y464V=π∫bax2dy=π64∫40y4dy=π64[y55]40=π64[455−0]=π64[10245]=16π5 unit3
Diagram 5 shows two curves x=√y and x=y28 are intersecting at point A. The straight line AB is parallel to the x-axis.

Find
(a) the equation of the straight line AB, [2 marks]
(b) area, in unit2, of the shaded region, [3 marks]
(c) the volume generated, in term of π, when the region P, which bounded by the curve x=y28 , the straight line AB and the y-axis, is revolved 360∘ about the y-axis. [3 marks]
Solution:
(a)
x=√y ….. (1)x=y28 ….. (2)Substitute (1) into (2),√y=y28(y12)2=(y28)2y=y46464=y4yy3=64y=4From (1),x=√4=2Point A=(2, 4)Equation of the straight line AB is y=4.
(b)
x=√y⇒y=x2x=y28⇒y=√8xArea of the shaded region=∫20(y1−y2)dx=∫20(√8x12−x2)dx=[√8x3232−x33]20=[2×2(2)12x323−x33]20=4(2)12(2)323−(2)33−0=163−83=83 unit2
(c)
x=y28⇒x2=y464V=π∫bax2dy=π64∫40y4dy=π64[y55]40=π64[455−0]=π64[10245]=16π5 unit3