SPM 2021 Add Maths Trial Paper (Selangor) – Paper 1 (Question 15)

Question 15:
Diagram 6 shows a triangle ABC. Given that the area of triangle ABC is 21 cm² and ∠BAC is obtuse angle.
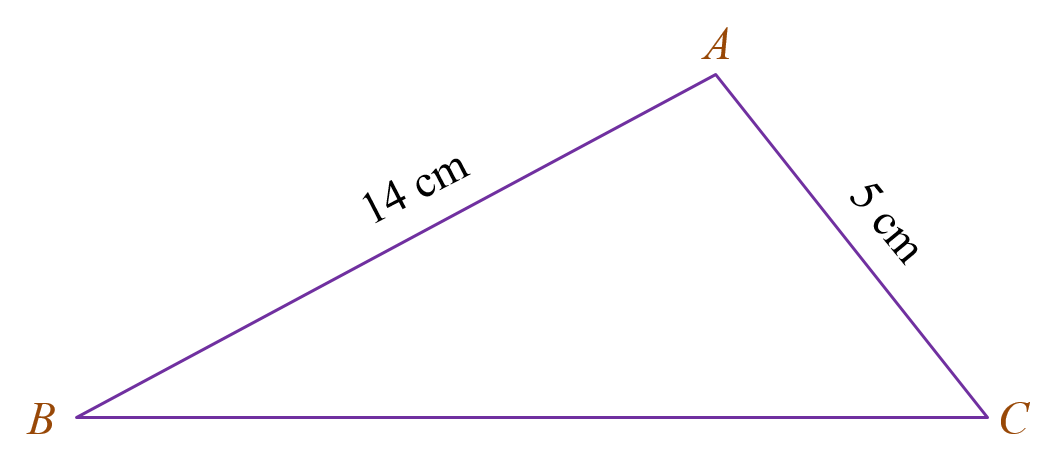Diagram 6

Find
(a) ∠BAC, [3 marks]
(b) the length of BC, in cm, [2 marks]
(c) the length of the perpendicular line from A to BC. [3 marks]


Solution:
(a)

$\begin{array}{l}\text{Area of }\Delta =\frac{1}{2}ab\sin C\\ 21=\frac{1}{2}\left(14\right)\left(5\right)\sin \angle BAC\\ \sin \angle BAC=\frac{21\times 2}{14\times 5}\\ \sin \angle BAC=0.6\\ \angle BAC={36.87}^o\\ \text{Obtuse angle of }\angle BAC={180}^o-{36.87}^o\\ ={143.13}^o\end{array}$


(b)

$\begin{array}{l}B{C}^2={14}^2+5^2-2\left(14\right)\left(5\right)cos{143.13}^o\\ B{C}^2=221-\left(-112\right)\\ BC=\sqrt{333}\\ =18.248\text{ cm}\end{array}$


(c)

$\begin{array}{l}\text{Given that the area of }\Delta ABC=21{\text{ cm}}^{\text{2}}\\ \text{Perpendicular line from }A\text{ to }BC\text{ is the verticle height, }h\text{ of the }△\text{,}\\ \frac{1}{2}\times h\times 18.248=21\\ h=\frac{21\times 2}{18.248}\\ =2.302\text{ cm}\end{array}$