Question 8 (10 marks):
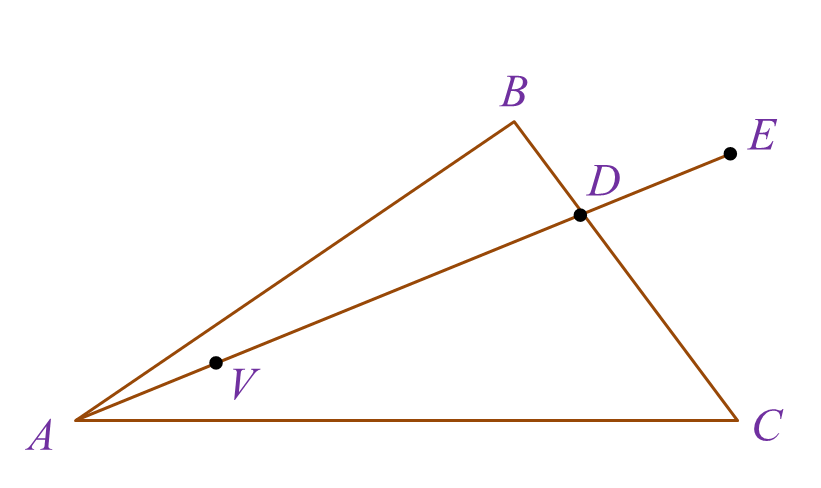
Diagram 5 shows a triangle ABC. The straight line AE intersects with the straight line BC at point D. Point V lies on the straight line AE.
Solution:
(a)(i)
(a)(ii)
(b)
(c)
Diagram 5 shows a triangle ABC. The straight line AE intersects with the straight line BC at point D. Point V lies on the straight line AE.

Solution:
(a)(i)
(a)(ii)
(b)
(c)
Question 9 (10 marks):
Use a graph to answer this question.
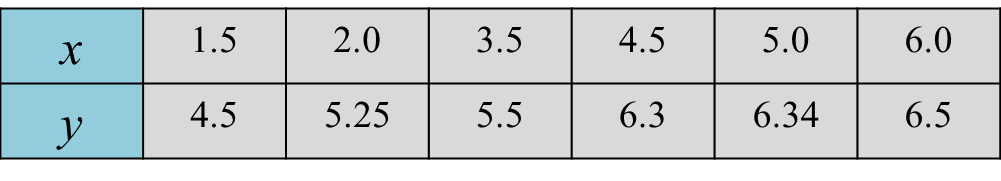
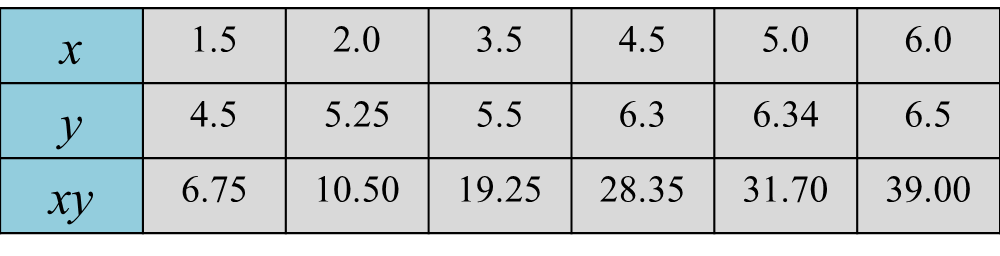
Table 1 shows the values of two variables, x and y, obtained from an experiment.
The variables x and y are related by the equation , where h and k are constants.
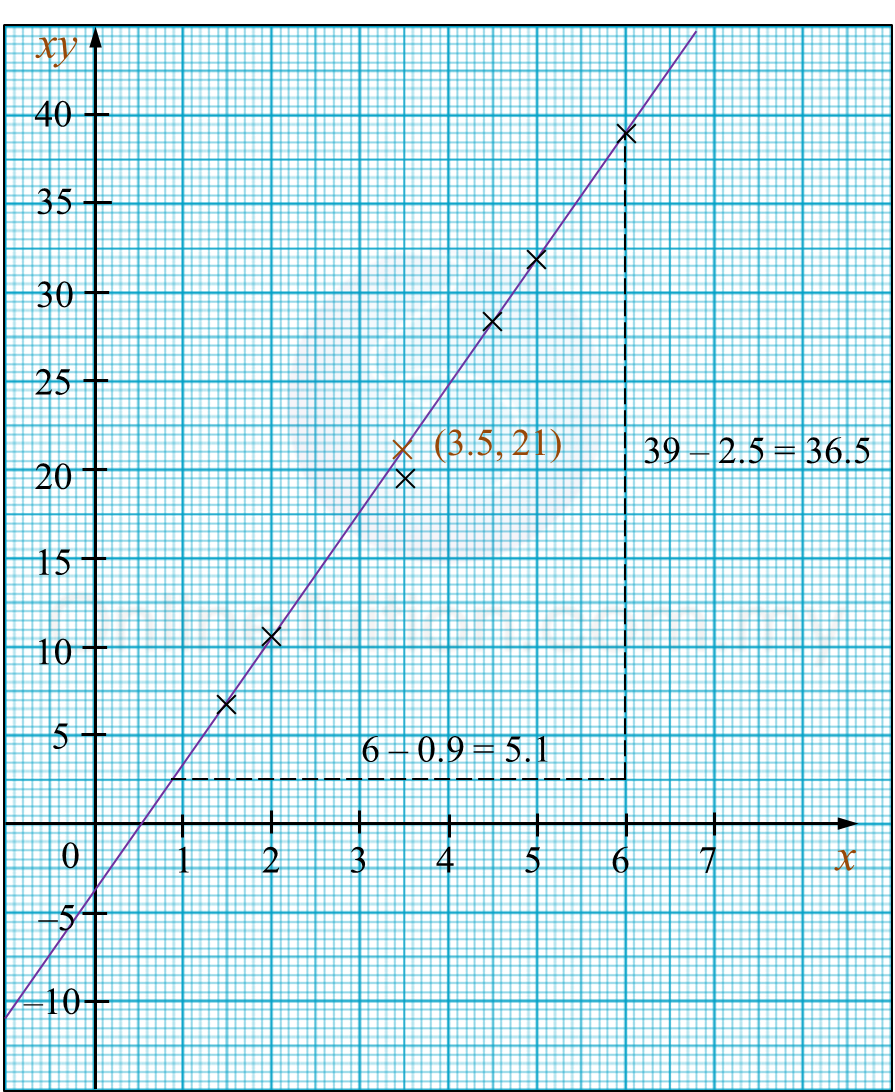
(a) Plot xy against x, using a scale of 2 cm to 1 unit on the x-axis and 2 cm to 5 units on the xy-axis.
Hence, draw the line of best fit.
(b) Using the graph in 9(a), find
(i) the value of h and of k,
(ii) the correct value of y if one of the values of y has been wrongly recorded during the experiment.
Solution:
(a)
(b)
(b)(i)
(b)(ii)
Use a graph to answer this question.
Table 1 shows the values of two variables, x and y, obtained from an experiment.
The variables x and y are related by the equation , where h and k are constants.

(a) Plot xy against x, using a scale of 2 cm to 1 unit on the x-axis and 2 cm to 5 units on the xy-axis.
Hence, draw the line of best fit.
(b) Using the graph in 9(a), find
(i) the value of h and of k,
(ii) the correct value of y if one of the values of y has been wrongly recorded during the experiment.
Solution:
(a)


(b)
(b)(i)
(b)(ii)