1.5.5 Circular Measure, Long Questions (Question 9)

Question 9:

In diagram 5, AOBDE, is a semicircle with centre O and has radius of 5cm. ABC is a right angle triangle.

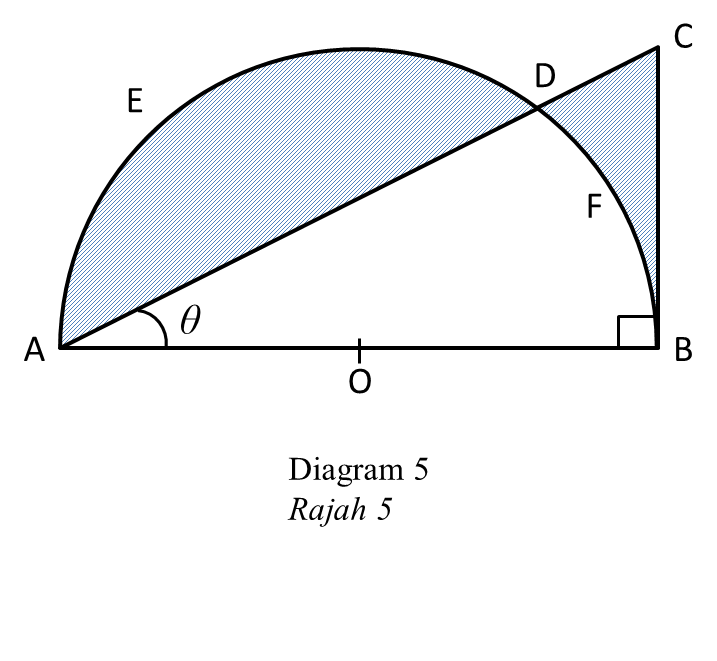
It is given that

\frac{A D}{D C} = 3.85 and D C = 2.31 cm .

[Use π = 3.142]

Calculate

(a) the value of θ, in radians, [2 marks]

(b) the perimeter, in cm, of the segment ADE, [3 marks]

Solution:

(a)

\begin{array}{l} Given \frac{A D}{D C} = 3.85 and D C = 2.31 cm \\ A D = 3.85 \times 2.31 = 8.8935 \\ ∴ A C = 8.8935 + 2.31 = 11.2035 cm \\ \cos θ = \frac{A B}{A C} = \frac{10}{11.2035} \\ θ = {26.80}^{o} \\ θ = {26.80}^{o} \times \frac{π}{180^{o}} = 0.4678 rad \end{array}

(b)

∠AOD = 3.142 – (0.4678 × 2)

= 2.206 rad

Length of arc AED = 5 × 2.206

= 11.03 cm

Therefore, perimeter of the segment ADE

= 11.03 + 8.8935

= 19.924 cm

(c)

BC = √ AC² – AB²

= √11.2035² – 10²

= 5.052 cm

\begin{array}{l} 2.206 rad \times \frac{180^{o}}{π} = {126.38}^{o} \\ ∠ B O D = 3.142 - 2.206 = 0.936 rad \\ Area of shaded region B C D F \\ = Area of △ A B C - Area of △ A O D - Area of segment O B D \\ = \frac{1}{2} (10) (5.052) - \frac{1}{2} (5) (5) (\sin 126.38) - \frac{1}{2} {(5)}^{2} (0.936) \\ = 25.26 - 10.06 - 11.7 \\ = 3.50 {cm}^{2} \end{array}