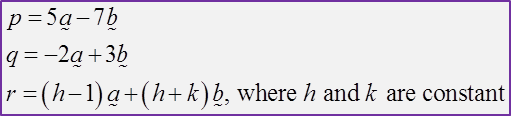Question 4:
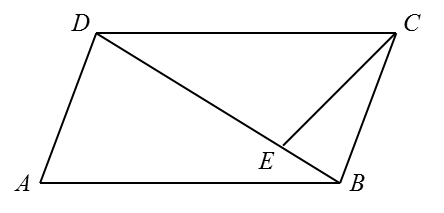
Given that →AB=7p˜, →AD=5q˜ and DE=3EB, express, in terms of p˜ and q˜.(a) →BD(b) →EC
Solution:
(a)
Note: for parallelogram,→AB=→DC=7p˜,→AD=→BC=5q˜.→BD=→BA+→AD→BD=−7p˜+5q˜
(b)
→DE=3→EB →EB →DE=13→EB:DE=1:3∴→EB=14→DB=14(−→BD)=14[−(−7p˜+5q˜ )]←From (a)=74p˜−54q˜
→EC=→EB+→BC→EC=74p˜−54q˜+5q˜→EC=74p˜+154q˜
Diagram below shows a parallelogram ABCD with BED as a straight line.
Given that →AB=7p˜, →AD=5q˜ and DE=3EB, express, in terms of p˜ and q˜.(a) →BD(b) →EC
Solution:
(a)
Note: for parallelogram,→AB=→DC=7p˜,→AD=→BC=5q˜.→BD=→BA+→AD→BD=−7p˜+5q˜
(b)
→DE=3→EB →EB →DE=13→EB:DE=1:3∴→EB=14→DB=14(−→BD)=14[−(−7p˜+5q˜ )]←From (a)=74p˜−54q˜
→EC=→EB+→BC→EC=74p˜−54q˜+5q˜→EC=74p˜+154q˜
Question 5:
Use the above information to find the values of h and k when r = 2p – 3q.
Solution:
r=2p−3q(h−1)a˜+(h+k)b˜=2(5a˜−7b˜)−3(−2a˜+3b˜)(h−1)a˜+(h+k)b˜=10a˜−14b˜+6a˜−9b˜(h−1)a˜+(h+k)b˜=16a˜−23b˜Comparing vector:h−1=16h=17h+k=−2317+k=−23k=−40
Use the above information to find the values of h and k when r = 2p – 3q.
Solution:
r=2p−3q(h−1)a˜+(h+k)b˜=2(5a˜−7b˜)−3(−2a˜+3b˜)(h−1)a˜+(h+k)b˜=10a˜−14b˜+6a˜−9b˜(h−1)a˜+(h+k)b˜=16a˜−23b˜Comparing vector:h−1=16h=17h+k=−2317+k=−23k=−40