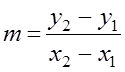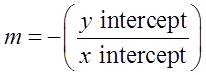\

7.4.1 Equations of Straight Lines (Part 1)

7.4.1 Axes Intercepts and Gradient
(A) Formula for gradient:
1. Gradient of the line joining (x1, yl) and (x2, y2) is:


2. Gradient of the line with knowing x–intercept and y–intercept is:


3. The gradient of the straight line joining P and Q is equal to the tangent of angle θ, where θ is the angle made by the straight line PQ and the positive direction of the x-axis.




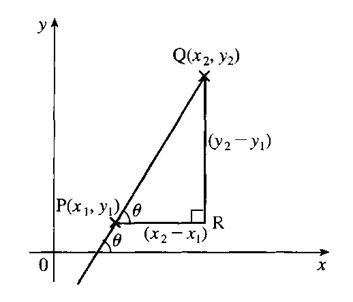
(B) Collinear points
The gradient of a straight line is always constant i.e.the gradient of AB is equal to the gradient of BC.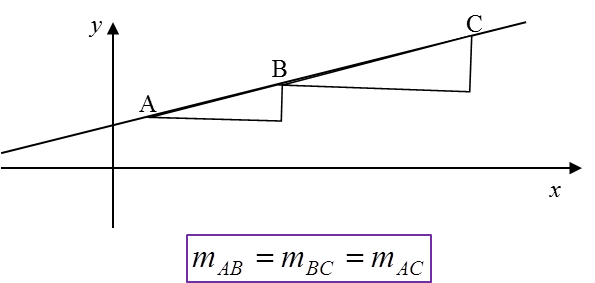


Example 1:
The gradient of the line passing through point (k, 1 – k) and point (–3k, –3) is 5.  Find the value of k.

Solution:
Gradient,  m = y 2 − y 1 x 2 − x 1 − 3 − ( 1 − k ) − 3 k − k = 5 − 3 − 1 + k − 4 k = 5 − 4 + k = − 20 k 21 k = 4 k = 4 21


Example 2:
Based on the diagram below, find the gradient of the line.
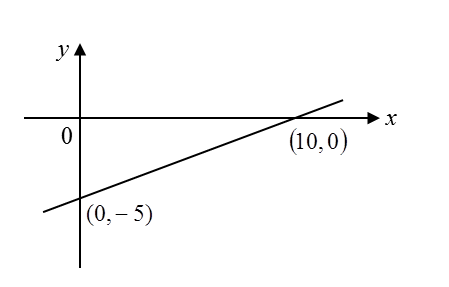
Solution:
Gradient,  m = − ( y  intercept x  intercept ) = − ( − 5 10 ) = 1 2

Leave a Comment