If you are asked to sketch the graph of a quadratic function, you need to show
a. the shape of the graph
b. the maximum/minimum point of the graph
c. the x-intercept of the graph
d. the y-intercept of the graph
Example
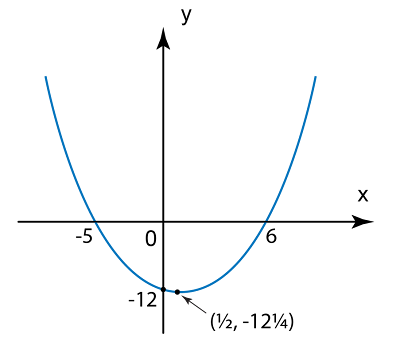
Sketch the curve of the quadratic function f(x)=x2−x−12
Answer:
The shape of the graph
Since the coefficient of x2 is positive, hence the graph is a U shape parabola with a minimum point.
The minimum point of the graph
By completing the square
f(x)=x2−x−12f(x)=x2−x+(12)2−(12)2−12f(x)=(x−12)2−14−12f(x)=(x−12)2−1214Minimum point = (12,−1214)
For y-intercept, x = 0
f(0)=(0)2−(0)−12=−12
For x-intercept, f(x) = 0
f(x)=x2−x−120=x2−x−12(x+5)(x−6)=0x=−5 or x=6
Sketch the curve of the quadratic function f(x)=x2−x−12
Answer:
The shape of the graph
Since the coefficient of x2 is positive, hence the graph is a U shape parabola with a minimum point.
The minimum point of the graph
By completing the square
f(x)=x2−x−12f(x)=x2−x+(12)2−(12)2−12f(x)=(x−12)2−14−12f(x)=(x−12)2−1214Minimum point = (12,−1214)
For y-intercept, x = 0
f(0)=(0)2−(0)−12=−12
For x-intercept, f(x) = 0
f(x)=x2−x−120=x2−x−12(x+5)(x−6)=0x=−5 or x=6
Suggested Video
Graphs of Quadratic Function - khanacademyAlgebra - Quadratic Functions (Parabolas) - yaymath