2.12.5 Quadratic Functions, SPM Practice (Paper 1)

Question 13:
$\text{Show that }6x-6-2k{x}^2=x^2\text{ has no real roots if }k>\frac{1}{4}.$

Solution:

Question 14:
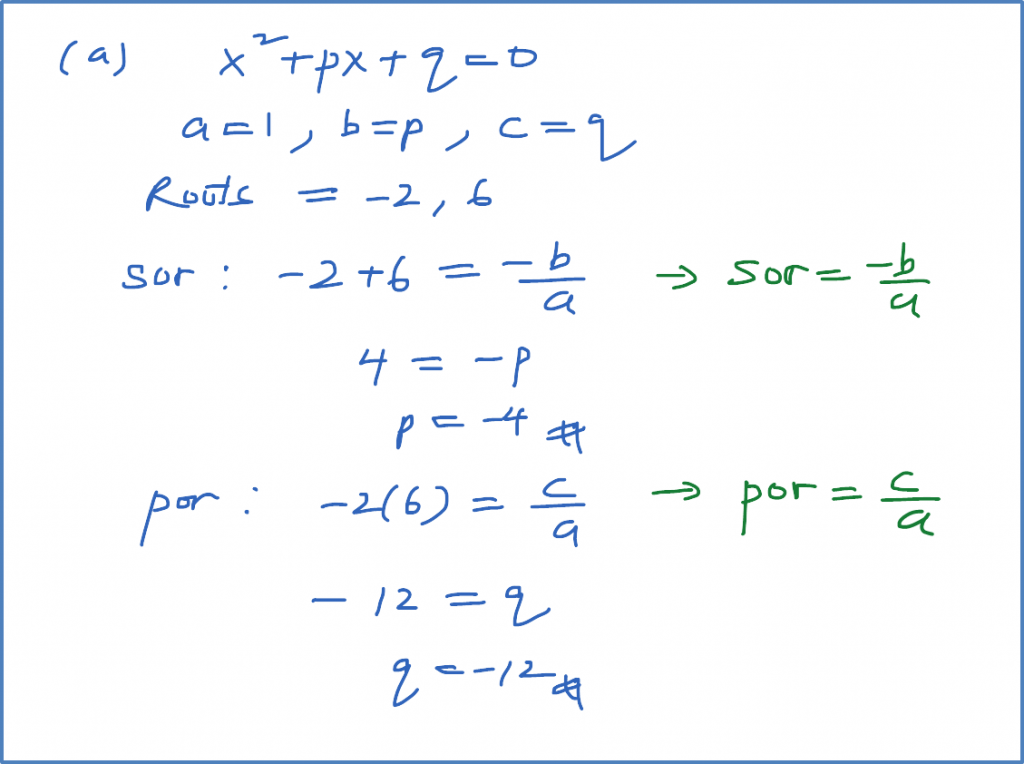
The quadratic equation $x^2+px+q=0$ has roots –2 and 6. Find
(a) the value of p and of q,
(b) the range of values of r for which the equation $x^2+px+q=r$ has no real roots.

Solution:

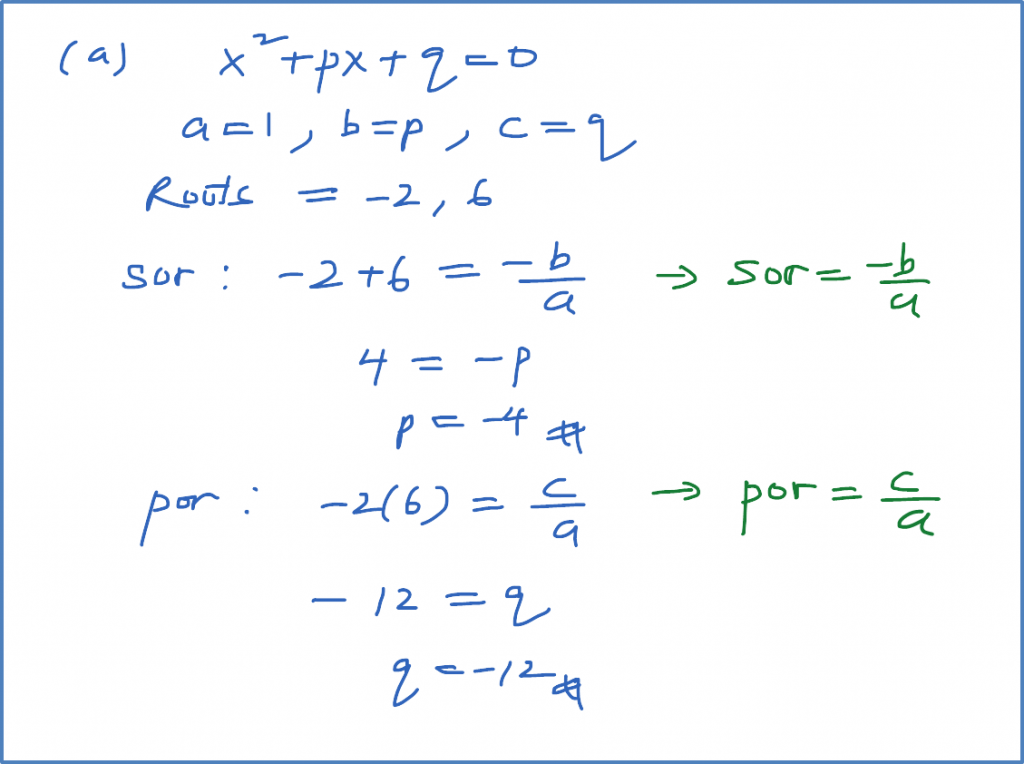
Question 15:
The straight line y = 5x – 1 does not intersect with the curve y = 2x² + x + h.
Find the range of values of h.

Solution:
$\begin{array}{l}y=5x-1\mathrm{\dots \dots }\text{ (1)}\\ y=2x^2+x+h\mathrm{\dots \dots }\text{ (2)}\\ \text{Substitute (1) into (2),}\\ 5x-1=2x^2+x+h\\ 2x^2+x+h-5x+1=0\\ 2x^2-4x+h+1=0\\ \\ b^2-4ac<0\\ {\left(-4\right)}^2-4\left(2\right)\left(h+1\right)<0\\ 16-8h-8<0\\ 8<8h\\ h>1\end{array}$

Question 16:
Find the range of values of p for which the equation $2x^2+5x+3-p=0$ has two real distinct roots.

Solution: