2.12.3 Quadratic Functions, SPM Practice (Paper 1)

Question 7:
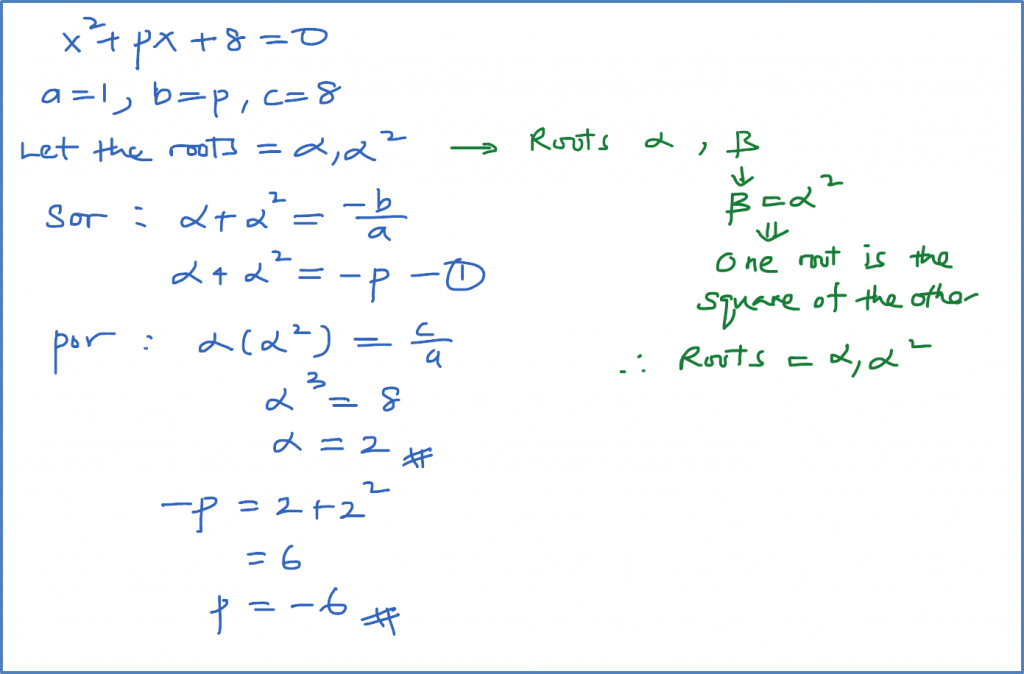
Find the value of p if one root of $x^2+px+8=0$ is the square of the other.

Solution:

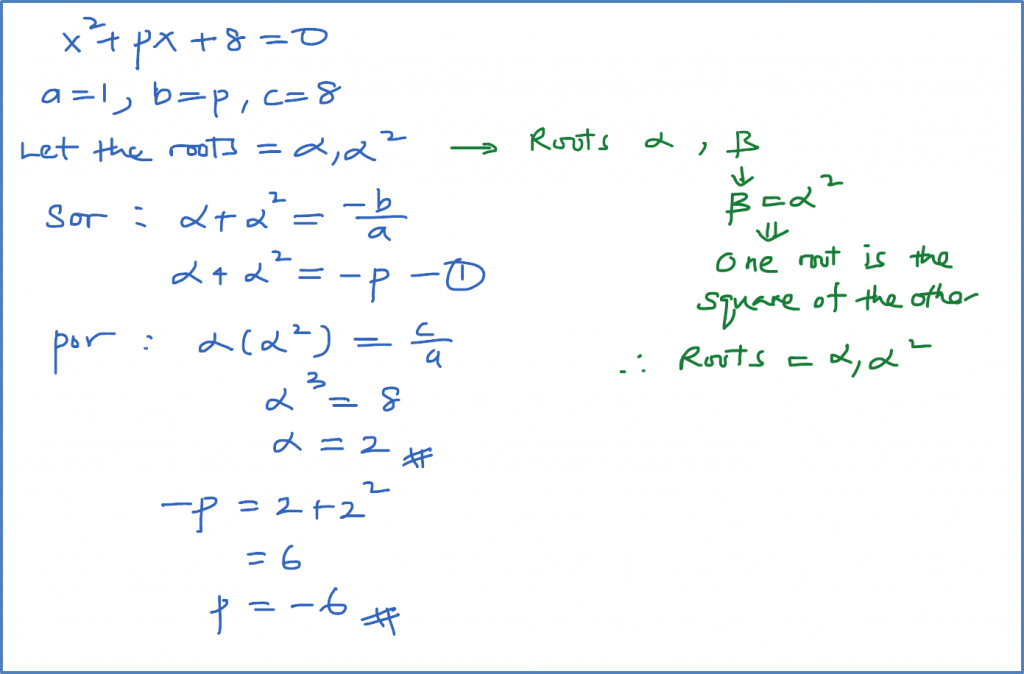
Question 8:
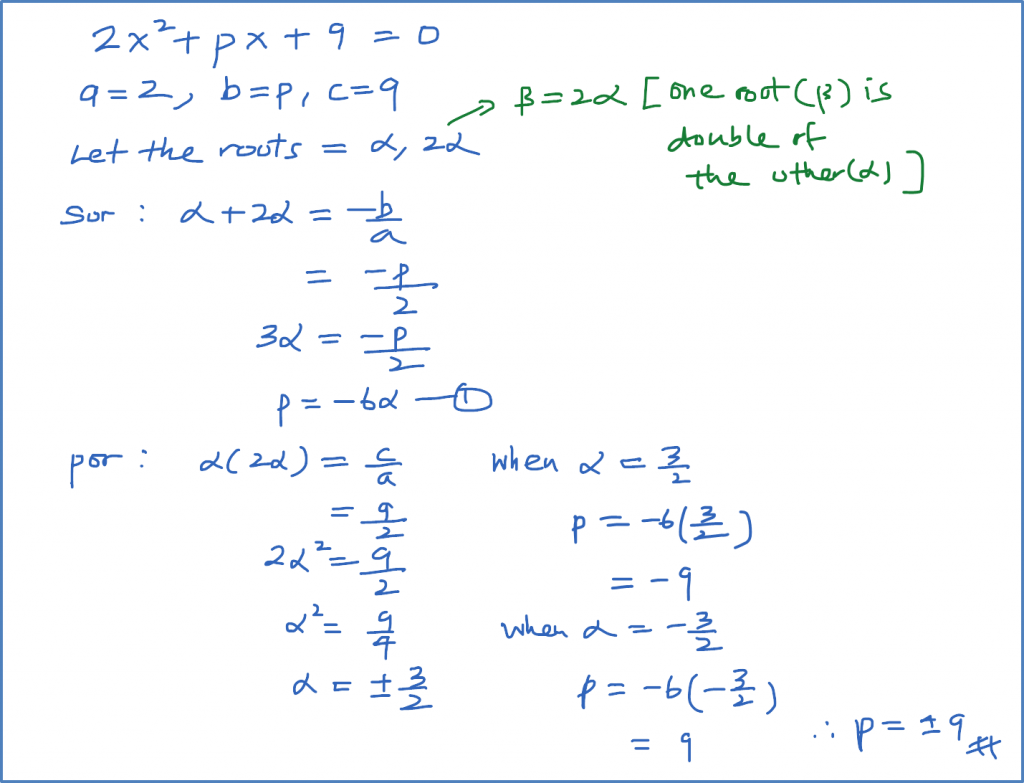
If one root of $2x^2+px+9=0$ is twice the other, find the values of p.

Solution:

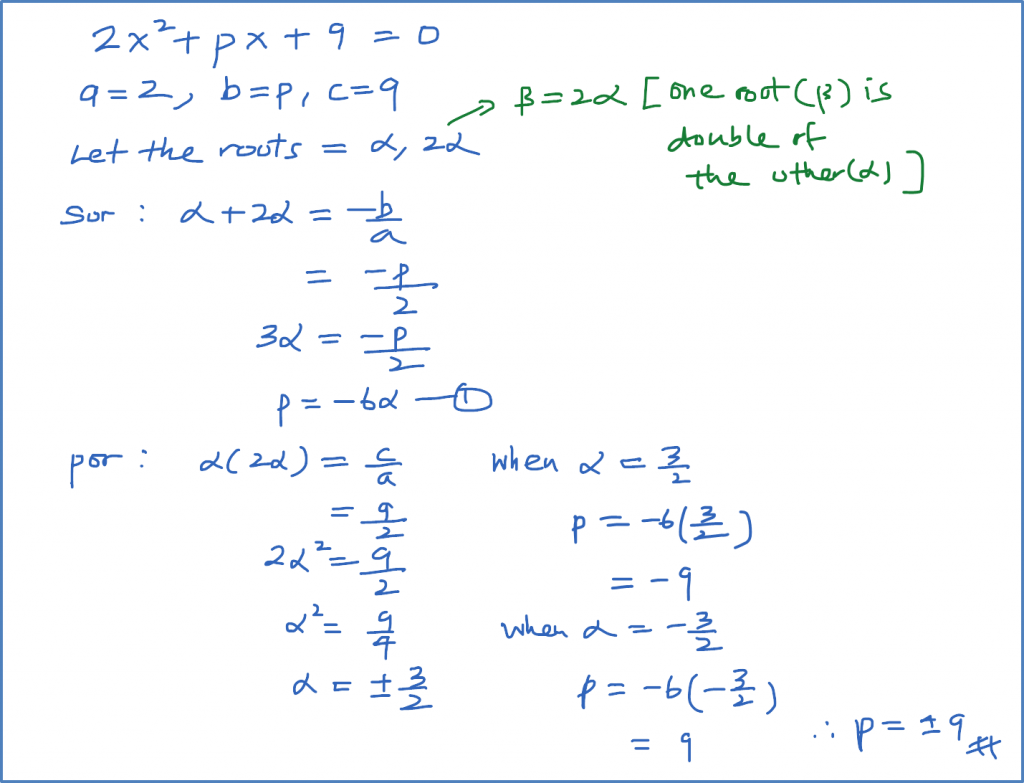
Question 9:
The roots of the equation $6x^2+hx+1=0\text{ are }\alpha \text{ and }\beta \text{, whereas 3}\alpha \text{ and 3}\beta$ are the roots of the equation $2x^2-x+k=0$ . Find the value of h and k.

Solution:
$\begin{array}{l}6x^2+hx+1=0\\ a=6,b=h,c=1\\ \text{Roots}=\alpha ,\beta \\ \text{sor}:\\ \alpha +\beta =-\frac{b}{a}\\ \alpha +\beta =-\frac{h}{6}\mathrm{\dots \dots \dots }\left(1\right)\\ por:\\ \alpha \beta =\frac{c}{a}\\ \alpha \beta =\frac{1}{6}\mathrm{\dots \dots \dots }\left(2\right)\\ \\ 2x^2-x+k=0\\ a=2,b=-1,c=k\\ \text{Roots}=3\alpha ,3\beta \\ \text{sor}:\\ 3\alpha +3\beta =-\frac{b}{a}\\ 3\left(\alpha +\beta \right)=-\frac{\left(-1\right)}{2}\\ \alpha +\beta =\frac{1}{6}\mathrm{\dots \dots \dots }\left(3\right)\\ por:\\ 3\alpha \left(3\beta \right)=\frac{c}{a}\\ 9\alpha \beta =\frac{k}{2}\\ k=18\alpha \beta \mathrm{\dots \dots \dots }\left(4\right)\\ \\ \text{Substitute }\left(3\right)\text{ into }\left(1\right)\\ \alpha +\beta =-\frac{h}{6}\\ \frac{1}{6}=-\frac{h}{6}\\ h=-1\\ \\ \text{Substitute }\left(2\right)\text{ into }\left(4\right)\\ k=18\alpha \beta \\ k=18\left(\frac{1}{6}\right)\\ k=3\end{array}$