1.7.3 Function, SPM Practice (Long Question)

Question 5:
In diagram below, the function g maps set P to set Q and the function h maps set Q to set R.

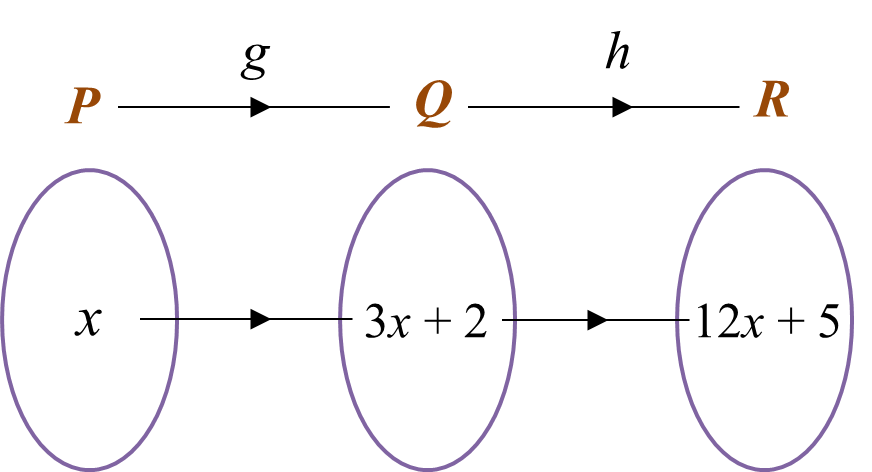
Find
(a) in terms of x, the function
(i) which maps set Q to set P,
(ii) h(x).
(b) the value of x such that gh(x) = 8x + 1.

Solution:
(a)(i)

\begin{array}{l} g (x) = 3 x + 2 \\ Let g^{- 1} (x) = y \\ g (y) = x \\ 3 y + 2 = x \\ y = \frac{x - 2}{3} \\ g^{- 1} (x) = \frac{x - 2}{3} \end{array}

(a)(ii)

\begin{array}{l} h g (x) = 12 x + 5 \\ h (3 x + 2) = 12 x + 5 \to g (x) = 3 x + 2 \\ Let u = 3 x + 2 \\ x = \frac{u - 2}{3} \\ h (u) = 12 (\frac{u - 2}{3}) + 5 \\ = 4 u - 8 + 5 \\ = 4 u - 3 \\ h (x) = 4 x - 3 \end{array}

(b)

\begin{array}{l} g h (x) = g (4 x - 3) \\ = 3 (4 x - 3) + 2 \\ = 12 x - 9 + 2 \\ = 12 x - 7 \\ 12 x - 7 = 8 x + 1 \\ 4 x = 8 \\ x = 2 \end{array}

Question 6:

Given that f : x \to \frac{5 x}{m x - 3}, x \neq \frac{3}{2} and g : x \to 3 - 4 x . Find

(a) the value of m, [2 marks]

(b) gf^-1(–2), [3 marks]

Solution:

(a)

\begin{array}{l} m x - 3 = 0 \\ m (\frac{3}{2}) - 3 = 0 \\ m = 3 \times \frac{2}{3} = 2 \end{array}

(b)

\begin{array}{l} Let y = \frac{5 x}{2 x - 3} \\ 2 x y - 3 y = 5 x \\ 2 x y - 5 x = 3 y \\ x (2 y - 5) = 3 y \\ x = \frac{3 y}{2 y - 5} \\ f^{- 1} (y) = \frac{3 y}{2 y - 5} \\ f^{- 1} (x) = \frac{3 x}{2 x - 5} \\ f^{- 1} (- 2) = \frac{3 (- 2)}{2 (- 2) - 5} = \frac{2}{3} \end{array}

\begin{array}{l} g f^{- 1} (- 2) = g (\frac{2}{3}) \\ = 3 - 4 (\frac{2}{3}) \\ = \frac{1}{3} \end{array}

(c)

hg (x) = 12x + 5

h [g(x)] = 12x + 5

h (3 – 4x) = 12x + 5

Let u = 3 – 4x

\begin{array}{l} x = \frac{3 - u}{4} \\ h (u) = 12 (\frac{3 - u}{4}) + 5 \\ h (u) = 9 - 3 u + 5 \\ h (u) = 14 - 3 u \\ h (x) = 14 - 3 x \end{array}