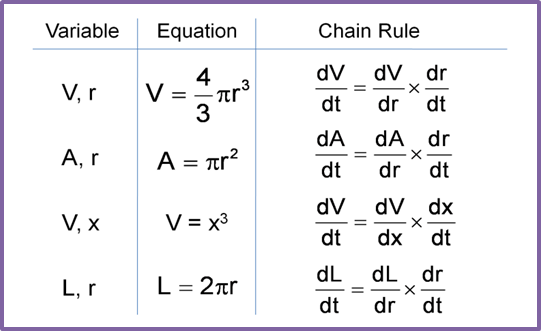
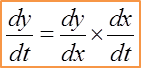(A) Related Rates of Change
Solution:
y=4x+3x=4x+3x−1dydx=4−3x−2=4−3x2dydt=dydx×dxdt2=(4−3x2)×dxdtwhen x=32=(4−332)×dxdt2=113×dxdtdxdt=611 unit s−1
1. If two variables x and y are connected by the equation y = f(x)
Notes:
If x changes at the rate of 5 cms -1 ⇒
dxdt=5
Decreases/leaks/reduces Þ NEGATIVES values!!!Example 1 (Rate of change of y and x)
Two variables, x and y are related by the equation
y=4x+3x
. Given that y increases at a constant rate of 2 units per second, find the rate of change of x when x = 3.Solution:
y=4x+3x=4x+3x−1dydx=4−3x−2=4−3x2dydt=dydx×dxdt2=(4−3x2)×dxdtwhen x=32=(4−332)×dxdt2=113×dxdtdxdt=611 unit s−1
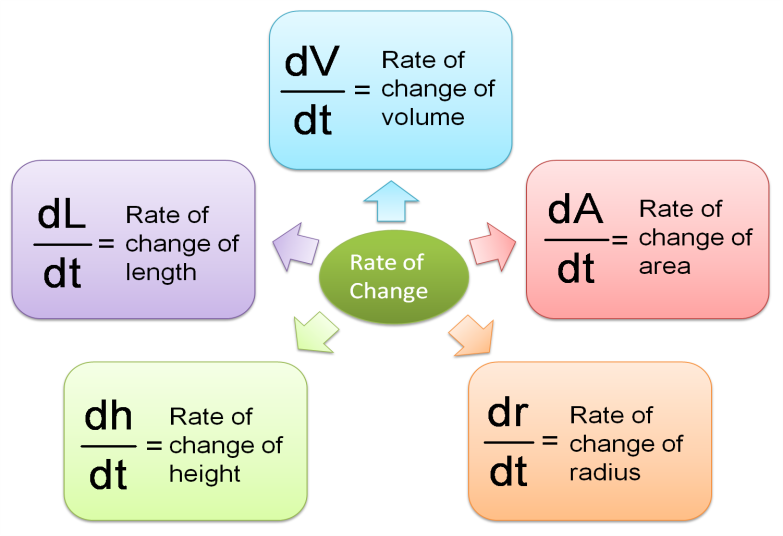
(B) Rates of Change of Volume, Area, Radius, Height and Length
(C) Rate of Change of Any Combination of Two Variables