Question 3:
Solution:
(a)
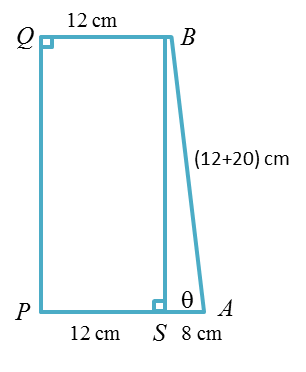
Diagram below shows two circles. The larger circle has centre A and radius 20 cm. The smaller circle has centre B and radius 12 cm. The circles touch at point R. The straight line PQ is a common tangent to the circles at point P and point Q.
[Use π = 3.142]
Given that angle PAR = θ radians,
(a) show that θ = 1.32 ( to two decimal places),
(b) calculate the length, in cm, of the minor arc QR,
(c) calculate the area, in cm2, of the shaded region.
Solution:
(a)
Question 4:
Diagram below shows a sector QPR with centre P and sector POQ, with centre O.
 It is given that OP = 17 cm and PQ = 8.8 cm.
It is given that OP = 17 cm and PQ = 8.8 cm.
[Use π = 3.142]
Calculate
(a) angle OPQ, in radians,
(b) the perimeter, in cm, of sector QPR,
(c) the area, in cm2, of the shaded region.
Solution:
Diagram below shows a sector QPR with centre P and sector POQ, with centre O.
 It is given that OP = 17 cm and PQ = 8.8 cm.
It is given that OP = 17 cm and PQ = 8.8 cm.[Use π = 3.142]
Calculate
(a) angle OPQ, in radians,
(b) the perimeter, in cm, of sector QPR,
(c) the area, in cm2, of the shaded region.
Solution: