Question 3:
Solution:
(a)
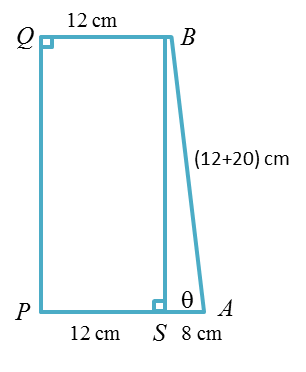
In △BSAcosθ=832=14 θ=1.32 rad (2 d.p.)
(b)
(c)
PQ=√322−82=30.98 cm
Diagram below shows two circles. The larger circle has centre A and radius 20 cm. The smaller circle has centre B and radius 12 cm. The circles touch at point R. The straight line PQ is a common tangent to the circles at point P and point Q.
[Use π = 3.142]
Given that angle PAR = θ radians,
(a) show that θ = 1.32 ( to two decimal places),
(b) calculate the length, in cm, of the minor arc QR,
(c) calculate the area, in cm2, of the shaded region.
Solution:
(a)

In △BSAcosθ=832=14 θ=1.32 rad (2 d.p.)
(b)
Angle QBR = 3.142 – 1.32 = 1.822 rad
Length of minor arc QR
= 12 × 1.822
= 21.86 cm(c)
PQ=√322−82=30.98 cm
Area of the shaded region
= Area of trapezium PQBA– Area of sector QBR – Area of sector PAR
= ½ (12 + 20) (30.98) – ½ (12)2 (1.822) – ½ (20)2(1.32)
= 495.68 – 131.18 – 264
= 100.5 cm2
Question 4:
Diagram below shows a sector QPR with centre P and sector POQ, with centre O.
 It is given that OP = 17 cm and PQ = 8.8 cm.
It is given that OP = 17 cm and PQ = 8.8 cm.
[Use π = 3.142]
Calculate
(a) angle OPQ, in radians,
(b) the perimeter, in cm, of sector QPR,
(c) the area, in cm2, of the shaded region.
Solution:
(a)∠OPQ=∠OQPx+x+30=180 2x=150 x=75∠OPQ=75×3.142180 =1.3092 radians
(b)Length of arc QR=rθ =8.8×1.3092 =11.52 cmPerimeter of sector QPR=11.52+8.8+8.8=29.12 cm
(c)30o=30×3.142180=0.5237 radArea of segment PQ=12r2(θ−sinθ)=12×172×(0.5237−sin30)=12×289×(0.5237−0.5)=3.4247 cm2Area of sector QPR=12r2θ=12×8.82×1.3092=50.692 cm2Area of shaded region=3.4247+50.692=54.1167 cm2
Diagram below shows a sector QPR with centre P and sector POQ, with centre O.
 It is given that OP = 17 cm and PQ = 8.8 cm.
It is given that OP = 17 cm and PQ = 8.8 cm.[Use π = 3.142]
Calculate
(a) angle OPQ, in radians,
(b) the perimeter, in cm, of sector QPR,
(c) the area, in cm2, of the shaded region.
Solution:
(a)∠OPQ=∠OQPx+x+30=180 2x=150 x=75∠OPQ=75×3.142180 =1.3092 radians
(b)Length of arc QR=rθ =8.8×1.3092 =11.52 cmPerimeter of sector QPR=11.52+8.8+8.8=29.12 cm
(c)30o=30×3.142180=0.5237 radArea of segment PQ=12r2(θ−sinθ)=12×172×(0.5237−sin30)=12×289×(0.5237−0.5)=3.4247 cm2Area of sector QPR=12r2θ=12×8.82×1.3092=50.692 cm2Area of shaded region=3.4247+50.692=54.1167 cm2
